Difference between revisions of "Closure depth"
Dronkers J (talk | contribs) |
Dronkers J (talk | contribs) |
||
| Line 1: | Line 1: | ||
| − | |||
{{Review | {{Review | ||
| Line 5: | Line 4: | ||
|AuthorID=120 | |AuthorID=120 | ||
}} | }} | ||
| + | |||
| + | |||
| + | |||
| + | The well-known Hallermeier-equation<ref name="Hallermeier 1981">Hallermeier, R. J. (1981). A Profile Zonation for Seasonal Sand Beaches from Wave Climate. Coastal Engineering, Vol. 4, 253-277.</ref> characterizes the depth of closure by significant [[waves]] occurring 12 hours in a given year. Since a clear potential exists to expand this definition into a more detailed contribution, such an attempt is presented below. It is recommended to read this article in conjunction with the article [[Shoreface profile]]. | ||
| + | |||
{{Definition|title=Closure depth | {{Definition|title=Closure depth | ||
| − | |definition= | + | |definition= The inner depth of closure (DoC) marks the transition from upper to lower shoreface, corresponding to the most landward depth seaward of which there is no significant change in bottom elevation during a given time interval<ref name = "kraus et al 1998">Kraus, N. C., Larson, M. and Wise, R. A. (1998) Depth of Closure in Beach-fill Design, Coastal Engineering Technical Note CETN II-40, 3/98, U.S. Army Engineer Waterways Experiment Station, Vicksburg, MS.</ref>. <br> |
| + | The outer depth of closure marks the transition from lower shoreface to continental shelf, and corresponds to the depth where the influence of wave action on cross-shore sediment transport is on average insignificant compared to other influences. }} | ||
| − | + | The definition of the inner depth of closure given by Krauss et al. (1998)<ref name = "kraus et al 1998"/> is general in that it applies to the open coast where nearshore [[waves]] and wave induced [[currents]] are the dominant sediment-transporting mechanisms, as well as to non-wave dominated locations, such as the beach adjacent to a long jetty, at which sediment may be jetted offshore by a-large [[rip current]] or an ebb [[tide|tidal]] shoal where the tidal current is a major contributor to the sediment-transporting processes. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | Hallermeier (1981, 1983)<ref name="Hallermeier 1981"/><ref name="Hallermeier 1983">Hallermeier, R. J. (1983). Sand Transport Limits in Coastal Structure Design, Proceedings, Coastal Structures ’83, American Society of Civil Engineers, pp. 703-716.</ref> defined three profile zones, i.e. a littoral zone which is usually called upper shoreface, a shoal or buffer zone which is usually called lower shoreface and an [[offshore zone]] or shelf zone. This partition defines two closure depths, namely: | |
| + | * an “inner” (closer to shore) closure depth <math>h_{in}</math> at the seaward limit of the upper shoreface, and | ||
| + | * an “outer” or “lower” (further from shore) closure depth <math>h_{out}</math> at the seaward limit of the lower shoreface. | ||
| − | < | + | Hallermeier (1981)<ref name="Hallermeier 1981"/> derived for the inner closure depth the formula |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | where <math> | + | <math>h_{in} = 2.28 H_{12h/y}- 68.5 (\Large\frac{ H_{12h/y}^2}{g T_{12h/y}^2}\normalsize), \qquad (1)</math> |
| + | |||
| + | where <math>H_{12h/y}</math> is the effective [[wave height]] just seaward of the breaker zone that is exceeded for 12 hours per year, i.e. the significant wave height with a probability of yearly exceedance of 0.137%, <math> T_{12h/y}</math> is the [[wave period]] associated with <math> H_{12h/y}</math>, and <math>g</math> is the acceleration of gravity. | ||
| + | |||
| + | For the outer closure depth Hallermeier (1983)<ref name="Hallermeier 1983"/> derived the formula | ||
| + | |||
| + | <math>h_{out} = 0.013 \,H_s T_s \, \sqrt{\Large\frac{g}{d_{50} (s-1)}\normalsize}, \qquad (2)</math> | ||
| + | |||
| + | where <math>H_s</math> and <math>T_s</math> are the significant wave height and period respectively, <math>d_{50}</math> is the median [[sediment]] diameter and <math>s</math> is the ratio of specific gravity of sand to that of fluid (about 2.65). As we can see <math>h_{in}</math> is solely related to the wave parameters, irrespective of typical sediment diameters, ranging between 0.16 and 0.42 mm, as validated and described by Hallermeier (1978, 1981)<ref name="Hallermeier 1978">Hallermeier, R. J. (1978). Uses for a calculated limit depth to beach erosion. Proceedings, 16th Coastal Engineering Conference, American Society of Civil Engineers, 1493- 15 12.</ref><ref name="Hallermeier 1981"/>, whereas <math>h_{out}</math> requires both hydrodynamic and sedimentological parameters. They are relevant for MLW ([[mean low water]]) conditions. | ||
| + | |||
| + | The theoretical background of the inner DoC <math>h_{in}</math> is based on its relation to the critical number <math>\Phi_{in}</math>, describing the threshold of erosive seabed agitation by wave action<ref name="Hallermeier 1978"/><ref name="Hallermeier 1981"/>: | ||
| + | |||
| + | <math>\Phi_{in} = \Large\frac {U_{b}^2}{(s-1)gh_{in}}\normalsize = 0.03 , \qquad (3)</math> | ||
| + | |||
| + | where <math>U_b</math> is the maximum horizontal wave-induced fluid velocity at water depth <math>h_{in}</math>. Using linear wave theory and the value of <math>s-1 = 1.65</math> for quartz sand in seawater, Hallermeier expressed this equation in the form of equation (1). The first term in that equation is directly proportional to wave height and is the main contributor to the DoC. The second term provides a small correction associated with the wave steepness. Equation (1) can be further generalized to incorporate other time scales by introducing into it a significant wave height exceeded 12 hours in a particular time interval. | ||
| + | |||
| + | Hallermeier based the outer DoC <math>h_{out}</math> on the critical mobility number <math>\Phi_{out}</math> corresponding to the peak near-bottom fluid kinetic energy per unit sediment grain volume sufficient to raise an immersed grain a distance <math>4d_{50}</math>: | ||
| + | |||
| + | <math>\Phi_{out}=\Large\frac {U_{b}^2}{(s-1)gd_{50}}\normalsize = 8 , \qquad (4)</math> | ||
| + | |||
| + | |||
| + | The concepts of <math>h_{in}</math> and <math>h_{out}</math> are illustrated in Fig. 1 by Krauss et al. (1998)<ref name = "kraus et al 1998">Kraus, N. C., Larson, M. and Wise, R. A. (1998) Depth of Closure in Beach-fill Design, Coastal Engineering Technical Note CETN II-40, 3/98, U.S. Army Engineer Waterways Experiment Station, Vicksburg, MS.</ref>. It shows the result of surveys on a profile survey line at the foot of 56th Street, Ocean City, Maryland. The envelope of recorded elevations (above and below the mean) in any profile survey over the 4-year interval of available data and the standard deviation of depths are plotted as functions of the distance offshore. The envelope tends to converge in the depth range of 5 to 6 m for this particular profile, seaward of which the profile elevations separate over the crests of the shoals. The depth in Fig. 1 is referenced to the National Geodetic Vertical Datum (NGVD). | ||
| + | Formation of the offshore shoals can be attributed to earlier coastal and geologic processes and not to offshore movement of sediment from the present beach. The convergence of the envelope landward of the offshore [[Shoal|shoals]] indicates that movement of sediment on the shoals is not directly related to sediment exchange on the nearshore profile. For the profile data shown in Fig. 1, corresponding to a 4-year time interval, <math>h_{in}</math> is approximately 5.5 m. | ||
| − | |||
| − | |||
{| style="text-align:center; font-size:85%; Width:525px; margin: 0 auto" | {| style="text-align:center; font-size:85%; Width:525px; margin: 0 auto" | ||
| − | |[[Image: | + | |[[Image:OceanCityProfiles.jpg|525px]] |
|- | |- | ||
|'''Fig. 1 Mean, envelope and standard deviation of profile survey elevations 56th Street, Ocean City, Maryland, from Krauss et al. (1998) <ref name = "kraus et al 1998"/>''' | |'''Fig. 1 Mean, envelope and standard deviation of profile survey elevations 56th Street, Ocean City, Maryland, from Krauss et al. (1998) <ref name = "kraus et al 1998"/>''' | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | Birkemeier (1985)<ref name ="Birkemeier 1985">Birkemeier, W. A. (1985). Field data on seaward limit of profile change. Journal of Waterway, Port, Coastal and Ocean Engineering 111(3), 598-602.</ref> refined the criterion (3) based on observed profile data, yielding a modified equation for <math>h_{in}</math>: | |
| − | Birkemeier (1985) <ref name ="Birkemeier 1985">Birkemeier, W. A. (1985). Field data on seaward limit of profile change. Journal of Waterway, Port, Coastal and Ocean Engineering 111(3), 598-602.</ref> | + | |
| − | < | + | <math>h_{in} = 1.75 H_{12h/y}- 57.9 (\Large\frac{ H_{12h/y}^2}{g T_{12h/y}^2}\normalsize). \qquad (5)</math> |
| − | < | ||
| − | |||
| − | |||
| − | |||
| − | <math>h_{in} = 1.75 H_{ | ||
| − | |||
| − | |||
| − | |||
It produces a smaller estimate of the depth of closure than the Hallermeier equation (1) for given wave conditions. | It produces a smaller estimate of the depth of closure than the Hallermeier equation (1) for given wave conditions. | ||
| − | Houston (1995) <ref name="Houston 1995">Houston, J. R. (1995). Beach-fill volume required to produce specified dry beach width, Coastal Engineering Technical Note 11-32, U.S. Army Engineer Waterways Experiment Station, Vicksburg, MS.</ref> simplified Birkemeier’s formulation using properties of a Pierson-Moskowitz wave spectrum ( | + | Udo et al. (2020)<ref>Udo, K., Ranasinghe, R. and Takeda, Y. (2020) An assessment of measured and computed depth of closure around Japan. Sci. Rep. 10, 2987</ref> tested equations (1) and (2) using observed profile data and observed values for <math>H_{12h/y}</math> and <math>T_{12h/y}</math> at different field sites around Japan. They concluded that the coefficients appearing in equation (1), although adequate in some cases<ref name=V19>Valiente, N.G., Masselink, G., Scott, T., Conley, D. and McCarroll, R.J. (2019) Role of waves and tides on depth of closure and potential for headland bypassing. Mar. Geol. 407, 60–75</ref>, are in principle location dependent, and hence, not generically applicable to coastal regions globally. |
| − | + | ||
| − | + | Based on a study of a macrotidal, embayed and high-energy beach of SW England, Valiente et al. (2019)<ref name=V19>Valiente, N.G., Masselink, G., Scott, T., Conley, D. and McCarroll, R.J. (2019) Role of waves and tides on depth of closure and potential for headland bypassing. Mar. Geol. 407, 60–75</ref> showed that in some cases tidal currents in combination with waves can considerably increase the inner closure depth <math>h_{in}</math>. This implies, for example, that sand transport around headlands can be more frequent than the estimate (1) suggests. | |
| − | + | ||
| − | + | Houston (1995)<ref name="Houston 1995">Houston, J. R. (1995). Beach-fill volume required to produce specified dry beach width, Coastal Engineering Technical Note 11-32, U.S. Army Engineer Waterways Experiment Station, Vicksburg, MS.</ref> simplified Birkemeier’s formulation using properties of a Pierson-Moskowitz wave spectrum (see [[Statistical description of wave parameters]]) and a modified exponential distribution of significant wave height over time to express the DoC in terms of mean annual significant wave height <math>\overline {H_{s}}</math> according to <math>h_{in} = 6.75 \overline {H_{s}}</math>. Following Houston’s approach, the Hallermeier equation can similarly be expressed in the form: | |
| − | + | ||
| − | <math>h_{in} = 8.9 \overline {H_{s}} </math><br> | + | <math>h_{in} = 8.9 \overline {H_{s}} . \qquad (6) </math><br> |
| − | + | ||
| − | + | Equation (6) has the advantage to incorporate only a single parameter to estimate the DoC without the need to determine the wave height and period exceeded 12 hours in a particular time interval. However, it is not applicable for estimating DoC for a particular storm event as discussed below.<p> | |
| − | + | In the original formulation of Hallermeier (1981)<ref name="Hallermeier 1981"/> and subsequent modifications, the DoC was defined based on the largest wave height exceeded 12 hours per year. This definition incorporates a time element, but the exact event associated with the value of wave height is ambiguous. Depending on changes in storm activity and wave conditions from year to year, the predicted DoC can vary substantially. In order to determine a representative value of the DoC based on this definition, wave conditions averaged over a period of several years must be employed. | |
| − | Equation | + | |
| − | In the original formulation of Hallermeier (1981) <ref name="Hallermeier 1981"/> and subsequent modifications, the DoC was defined based on the largest wave height exceeded 12 hours per year. This definition incorporates a time element, but the exact event associated with the value of wave height is ambiguous. Depending on changes in storm activity and wave conditions from year to year, the predicted DoC can vary substantially. In order to determine a representative value of the DoC based on this definition, wave conditions averaged over a period of several years must be employed. | + | |
| − | A useful extension of calculating the DoC based on average annual wave conditions is to relate the DoC to a particular time period of interest over which specific storm events or seasonal wave conditions occur. A similar “wave-by-wave” interpretation of the DoC was introduced by Kraus and Harikai (1983) <ref name="Kraus and Harikai (1983)">Kraus, N. C., and Harikai, S. (1983). Numerical model of the shoreline change at Oarai Beach, CoastaI Engineering 7(l), l-28.</ref>. In a wave-by-wave or event approach, the DoC can be associated with a ''recurrence frequency'' or return period for a particular storm. The return period should be associated with the wave height, not with the [[storm surge]]. | + | A useful extension of calculating the DoC based on average annual wave conditions is to relate the DoC to a particular time period of interest over which specific storm events or seasonal wave conditions occur. A similar “wave-by-wave” interpretation of the DoC was introduced by Kraus and Harikai (1983)<ref name="Kraus and Harikai (1983)">Kraus, N. C., and Harikai, S. (1983). Numerical model of the shoreline change at Oarai Beach, CoastaI Engineering 7(l), l-28.</ref>. In a wave-by-wave or event approach, the DoC can be associated with a ''recurrence frequency'' or return period for a particular storm. The return period should be associated with the wave height, not with the [[storm surge]]. |
==Related articles== | ==Related articles== | ||
| + | :[[Shoreface profile]] | ||
:[[Active coastal zone]] | :[[Active coastal zone]] | ||
| − | |||
:[[Bruun rule]] | :[[Bruun rule]] | ||
Revision as of 15:18, 12 June 2021
The well-known Hallermeier-equation[1] characterizes the depth of closure by significant waves occurring 12 hours in a given year. Since a clear potential exists to expand this definition into a more detailed contribution, such an attempt is presented below. It is recommended to read this article in conjunction with the article Shoreface profile.
Definition of Closure depth:
The inner depth of closure (DoC) marks the transition from upper to lower shoreface, corresponding to the most landward depth seaward of which there is no significant change in bottom elevation during a given time interval[2].
The outer depth of closure marks the transition from lower shoreface to continental shelf, and corresponds to the depth where the influence of wave action on cross-shore sediment transport is on average insignificant compared to other influences. This is the common definition for Closure depth, other definitions can be discussed in the article
|
The definition of the inner depth of closure given by Krauss et al. (1998)[2] is general in that it applies to the open coast where nearshore waves and wave induced currents are the dominant sediment-transporting mechanisms, as well as to non-wave dominated locations, such as the beach adjacent to a long jetty, at which sediment may be jetted offshore by a-large rip current or an ebb tidal shoal where the tidal current is a major contributor to the sediment-transporting processes.
Hallermeier (1981, 1983)[1][3] defined three profile zones, i.e. a littoral zone which is usually called upper shoreface, a shoal or buffer zone which is usually called lower shoreface and an offshore zone or shelf zone. This partition defines two closure depths, namely:
- an “inner” (closer to shore) closure depth [math]h_{in}[/math] at the seaward limit of the upper shoreface, and
- an “outer” or “lower” (further from shore) closure depth [math]h_{out}[/math] at the seaward limit of the lower shoreface.
Hallermeier (1981)[1] derived for the inner closure depth the formula
[math]h_{in} = 2.28 H_{12h/y}- 68.5 (\Large\frac{ H_{12h/y}^2}{g T_{12h/y}^2}\normalsize), \qquad (1)[/math]
where [math]H_{12h/y}[/math] is the effective wave height just seaward of the breaker zone that is exceeded for 12 hours per year, i.e. the significant wave height with a probability of yearly exceedance of 0.137%, [math] T_{12h/y}[/math] is the wave period associated with [math] H_{12h/y}[/math], and [math]g[/math] is the acceleration of gravity.
For the outer closure depth Hallermeier (1983)[3] derived the formula
[math]h_{out} = 0.013 \,H_s T_s \, \sqrt{\Large\frac{g}{d_{50} (s-1)}\normalsize}, \qquad (2)[/math]
where [math]H_s[/math] and [math]T_s[/math] are the significant wave height and period respectively, [math]d_{50}[/math] is the median sediment diameter and [math]s[/math] is the ratio of specific gravity of sand to that of fluid (about 2.65). As we can see [math]h_{in}[/math] is solely related to the wave parameters, irrespective of typical sediment diameters, ranging between 0.16 and 0.42 mm, as validated and described by Hallermeier (1978, 1981)[4][1], whereas [math]h_{out}[/math] requires both hydrodynamic and sedimentological parameters. They are relevant for MLW (mean low water) conditions.
The theoretical background of the inner DoC [math]h_{in}[/math] is based on its relation to the critical number [math]\Phi_{in}[/math], describing the threshold of erosive seabed agitation by wave action[4][1]:
[math]\Phi_{in} = \Large\frac {U_{b}^2}{(s-1)gh_{in}}\normalsize = 0.03 , \qquad (3)[/math]
where [math]U_b[/math] is the maximum horizontal wave-induced fluid velocity at water depth [math]h_{in}[/math]. Using linear wave theory and the value of [math]s-1 = 1.65[/math] for quartz sand in seawater, Hallermeier expressed this equation in the form of equation (1). The first term in that equation is directly proportional to wave height and is the main contributor to the DoC. The second term provides a small correction associated with the wave steepness. Equation (1) can be further generalized to incorporate other time scales by introducing into it a significant wave height exceeded 12 hours in a particular time interval.
Hallermeier based the outer DoC [math]h_{out}[/math] on the critical mobility number [math]\Phi_{out}[/math] corresponding to the peak near-bottom fluid kinetic energy per unit sediment grain volume sufficient to raise an immersed grain a distance [math]4d_{50}[/math]:
[math]\Phi_{out}=\Large\frac {U_{b}^2}{(s-1)gd_{50}}\normalsize = 8 , \qquad (4)[/math]
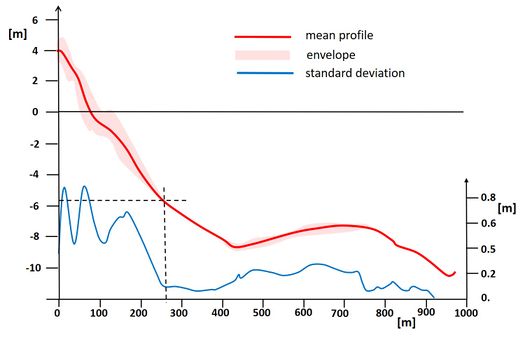
The concepts of [math]h_{in}[/math] and [math]h_{out}[/math] are illustrated in Fig. 1 by Krauss et al. (1998)[2]. It shows the result of surveys on a profile survey line at the foot of 56th Street, Ocean City, Maryland. The envelope of recorded elevations (above and below the mean) in any profile survey over the 4-year interval of available data and the standard deviation of depths are plotted as functions of the distance offshore. The envelope tends to converge in the depth range of 5 to 6 m for this particular profile, seaward of which the profile elevations separate over the crests of the shoals. The depth in Fig. 1 is referenced to the National Geodetic Vertical Datum (NGVD).
Formation of the offshore shoals can be attributed to earlier coastal and geologic processes and not to offshore movement of sediment from the present beach. The convergence of the envelope landward of the offshore shoals indicates that movement of sediment on the shoals is not directly related to sediment exchange on the nearshore profile. For the profile data shown in Fig. 1, corresponding to a 4-year time interval, [math]h_{in}[/math] is approximately 5.5 m.
|
| Fig. 1 Mean, envelope and standard deviation of profile survey elevations 56th Street, Ocean City, Maryland, from Krauss et al. (1998) [2] |
Birkemeier (1985)[5] refined the criterion (3) based on observed profile data, yielding a modified equation for [math]h_{in}[/math]:
[math]h_{in} = 1.75 H_{12h/y}- 57.9 (\Large\frac{ H_{12h/y}^2}{g T_{12h/y}^2}\normalsize). \qquad (5)[/math]
It produces a smaller estimate of the depth of closure than the Hallermeier equation (1) for given wave conditions. Udo et al. (2020)[6] tested equations (1) and (2) using observed profile data and observed values for [math]H_{12h/y}[/math] and [math]T_{12h/y}[/math] at different field sites around Japan. They concluded that the coefficients appearing in equation (1), although adequate in some cases[7], are in principle location dependent, and hence, not generically applicable to coastal regions globally.
Based on a study of a macrotidal, embayed and high-energy beach of SW England, Valiente et al. (2019)[7] showed that in some cases tidal currents in combination with waves can considerably increase the inner closure depth [math]h_{in}[/math]. This implies, for example, that sand transport around headlands can be more frequent than the estimate (1) suggests.
Houston (1995)[8] simplified Birkemeier’s formulation using properties of a Pierson-Moskowitz wave spectrum (see Statistical description of wave parameters) and a modified exponential distribution of significant wave height over time to express the DoC in terms of mean annual significant wave height [math]\overline {H_{s}}[/math] according to [math]h_{in} = 6.75 \overline {H_{s}}[/math]. Following Houston’s approach, the Hallermeier equation can similarly be expressed in the form:
[math]h_{in} = 8.9 \overline {H_{s}} . \qquad (6) [/math]
In the original formulation of Hallermeier (1981)[1] and subsequent modifications, the DoC was defined based on the largest wave height exceeded 12 hours per year. This definition incorporates a time element, but the exact event associated with the value of wave height is ambiguous. Depending on changes in storm activity and wave conditions from year to year, the predicted DoC can vary substantially. In order to determine a representative value of the DoC based on this definition, wave conditions averaged over a period of several years must be employed. A useful extension of calculating the DoC based on average annual wave conditions is to relate the DoC to a particular time period of interest over which specific storm events or seasonal wave conditions occur. A similar “wave-by-wave” interpretation of the DoC was introduced by Kraus and Harikai (1983)[9]. In a wave-by-wave or event approach, the DoC can be associated with a recurrence frequency or return period for a particular storm. The return period should be associated with the wave height, not with the storm surge.
Related articles
References
- ↑ 1.0 1.1 1.2 1.3 1.4 1.5 Hallermeier, R. J. (1981). A Profile Zonation for Seasonal Sand Beaches from Wave Climate. Coastal Engineering, Vol. 4, 253-277.
- ↑ 2.0 2.1 2.2 2.3 Kraus, N. C., Larson, M. and Wise, R. A. (1998) Depth of Closure in Beach-fill Design, Coastal Engineering Technical Note CETN II-40, 3/98, U.S. Army Engineer Waterways Experiment Station, Vicksburg, MS.
- ↑ 3.0 3.1 Hallermeier, R. J. (1983). Sand Transport Limits in Coastal Structure Design, Proceedings, Coastal Structures ’83, American Society of Civil Engineers, pp. 703-716.
- ↑ 4.0 4.1 Hallermeier, R. J. (1978). Uses for a calculated limit depth to beach erosion. Proceedings, 16th Coastal Engineering Conference, American Society of Civil Engineers, 1493- 15 12.
- ↑ Birkemeier, W. A. (1985). Field data on seaward limit of profile change. Journal of Waterway, Port, Coastal and Ocean Engineering 111(3), 598-602.
- ↑ Udo, K., Ranasinghe, R. and Takeda, Y. (2020) An assessment of measured and computed depth of closure around Japan. Sci. Rep. 10, 2987
- ↑ 7.0 7.1 Valiente, N.G., Masselink, G., Scott, T., Conley, D. and McCarroll, R.J. (2019) Role of waves and tides on depth of closure and potential for headland bypassing. Mar. Geol. 407, 60–75
- ↑ Houston, J. R. (1995). Beach-fill volume required to produce specified dry beach width, Coastal Engineering Technical Note 11-32, U.S. Army Engineer Waterways Experiment Station, Vicksburg, MS.
- ↑ Kraus, N. C., and Harikai, S. (1983). Numerical model of the shoreline change at Oarai Beach, CoastaI Engineering 7(l), l-28.
|