Difference between revisions of "Seawater intrusion and mixing in estuaries"
Dronkers J (talk | contribs) |
Dronkers J (talk | contribs) |
||
| (9 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | |||
| + | We describe in this article the physical processes involved in seawater intrusion and mixing in estuaries under the influence of tidal motion and explain some simple methods for deriving quantitative estimates. Several of the dispersion mechanisms discussed in this article are illustrated by dye experiments shown in [[Estuarine dispersion: dye experiments in the Eastern Scheldt scale model]]. | ||
| + | |||
==Introduction== | ==Introduction== | ||
| − | Estuaries are generally defined as semi-enclosed transition zones between river and sea. The intrusion of seawater in estuaries is mainly due to tides and | + | Estuaries are generally defined as semi-enclosed transition zones between river and sea. The intrusion of seawater in estuaries determines the salinity distribution. Salinity is usually expressed as the weight in grams of all dissolved salts per kilogram of water or alternatively according to the Practical Salinity Scale (see [[Salinity]]). The seawater intrusion limit is determined by the location in the estuary where the difference with river salinity is below a small number. Seawater intrusion is mainly due to tides and to the influence of the density difference between seawater and river water on currents and turbulence (this influence is often termed 'buoyancy effects', see the article [[Estuarine circulation]] for further details). It is counteracted by freshwater inflow from rivers, groundwater, and other sources. Seawater intrusion in estuaries is an important phenomenon to man and nature: it limits freshwater availability for human and agricultural use, and it determines the type of habitats and species that can develop in an estuarine environment. Besides, density driven currents and salinity play a role in estuarine turbidity and sedimentation processes. |
| − | The net effect of estuarine mixing processes during a tidal cycle on along-channel seawater intrusion or on net along-channel spreading (diffusion) of dissolved substances is generally called '''longitudinal dispersion'''. This net effect is described by a longitudinal dispersion coefficient <math>K_x</math>. The different mixing processes contributing to longitudinal dispersion are termed '''dispersion mechanisms'''. In well-mixed and partially mixed estuaries these mixing processes are dominantly induced by tidal motion; the net effect on seawater intrusion is called '''tidal longitudinal dispersion''' or simply '''tidal dispersion'''. | + | The net effect of estuarine mixing processes during a tidal cycle on along-channel seawater intrusion or on net along-channel spreading (diffusion) of dissolved substances is generally called '''longitudinal dispersion'''. This net effect is described by a longitudinal dispersion coefficient <math>K_x</math>. The different mixing processes contributing to longitudinal dispersion are termed '''dispersion mechanisms'''. In well-mixed and partially mixed estuaries these mixing processes are dominantly induced by tidal motion; the net effect on seawater intrusion is called '''tidal longitudinal dispersion''' or simply '''tidal dispersion'''. Longitudinal dispersion and the '''flushing time''' of an estuary are related concepts. The combined effect of all tidal dispersion processes on seawater intrusion is sometimes called 'tidal pumping'. Here we use the term 'tidal pumping' only for the fraction of outflowing estuarine water that is replaced by 'new' seawater at the estuarine mouth. |
| + | |||
| + | Seawater intrusion has a strong variability. At a fixed station, the salinity increases during high tide and is greatest at high water; the salinity decreases during low tide and is smallest at low tide. Besides, seawater intrusion is small with high river discharge and strong with low river discharge. Change in seawater intrusion with river discharge is more gradual than the variability due to flood and ebb. If the river discharge varies on a time scale greater than the flushing time of the estuary, the tide-averaged salinity distribution tends towards an equilibrium distribution. The longitudinal dispersion coefficient can be determined by measuring the salinity distribution under equilibrium conditions. This coefficient generally varies along the estuary and depends on the river discharge. | ||
| + | |||
| + | The dispersion processes that are responsible for seawater intrusion determine the flushing time as well as the '''residence time''' of dissolved substances in an estuary. This holds similarly for the residence time of micro-organisms that are carried along passively (not swimming, floating or settling) with the water. | ||
==Definitions and assumptions== | ==Definitions and assumptions== | ||
| − | The estuarine mouth is defined as the transition between zones of channeled tidal flow ( | + | The estuarine mouth is defined as the transition between zones of channeled tidal flow (tidal discharge mainly following the channel axis) and non-channeled flow (discharge deviated along the open coast). The estuarine head is defined here as the location where the seawater influence on the salinity is (almost) nil; this location depends on the river discharge. We are interested in the net effect of tidal mixing processes during a tidal cycle on seawater intrusion along the estuarine axis, defined as the <math>x</math>-axis (<math>x=0</math> at the estuarine mouth). This makes sense only if we assume that the salinity difference between the estuarine mouth and the estuarine head is much greater than salinity differences in lateral (<math>y</math>-axis) and vertical (<math>z</math>-axis) directions. Estuaries where salinity differences between bottom and surface are comparable to the salinity differences between estuarine mouth and estuarine head are dealt with in the article [[Salt wedge estuaries]]. The estuaries considered in this article are often designated as well-mixed or partially mixed estuaries. |
Successive tidal cycles are not identical. Determining the net effect of tidally induced mixing processes requires integration over a large number of tidal cycles. To prevent this complication, we assume that successive tidal cycles are sufficiently comparable (no extreme spring-neap variation) to enable the definition of a representative cyclic tide. This assumption will be used throughout this article. | Successive tidal cycles are not identical. Determining the net effect of tidally induced mixing processes requires integration over a large number of tidal cycles. To prevent this complication, we assume that successive tidal cycles are sufficiently comparable (no extreme spring-neap variation) to enable the definition of a representative cyclic tide. This assumption will be used throughout this article. | ||
| Line 13: | Line 20: | ||
==Random walk== | ==Random walk== | ||
| − | + | Under certain conditions, estuarine mixing processes can be described in terms of random walk. For simplicity we consider a tidal basin with small river inflow (river discharge much smaller than tidal discharges). Situations where river flow exerts a substantial influence on estuarine water motion are dealt with in the article [[Estuarine circulation]]. Water motion is described as the average motion of a very large number of individual water parcels. If individual water parcels follow identical paths during flood (inflow) and ebb (outflow) all seawater parcels entering the basin during flood will be expelled during ebb: no seawater intrusion will occur. In reality this will not happen; mixing processes ensure that individual water parcels follow different paths during flood and ebb. Water parcels move back and forth in a tidal basin for some time before finally leaving the estuary and being evacuated offshore. We call <math> T_x </math> the flushing time of water parcels in the tidal basin; it is defined as the average time it takes for a fluid parcel to travel from the upstream boundary of the estuary to the sea. During this time, water parcels also move in lateral and vertical directions, due to flow circulations and turbulent eddies. The time scale for vertical mixing, <math>T_z </math>, and the time scale for lateral mixing, <math>T_y </math>, are related to the vertical and lateral turbulent diffusion coefficients, <math>K_z </math> and <math>K_y </math>, by the relationships <math>T_z=D^2/K_z </math> and <math>T_y=B^2/K_y </math>, respectively. The time scales <math>T_y </math> and <math>T_z </math> indicate the time during which the motions of individual water parcels remain correlated; after a longer time they have lost the memory of their initial position <math>(y_0,z_0)</math> in the estuarine cross-section. The probability that after this time they will be in a cross-sectional position <math>(y,z)</math> from where their net displacement during the next tidal cycle will be in landward direction is then equal to the probability that they will be in a position where their net displacement will be in seaward direction, if the influence of river discharge is ignored. The cross-sectional mixing time <math>T_A </math> is approximately equal to the lateral mixing time <math>T_y</math>, if we assume that lateral mixing takes more time than vertical mixing; this is the case for most well-mixed or partially mixed wide estuaries. If <math>T_A</math> is much smaller than the longitudinal flushing time <math>T_x</math> of water parcels in the estuary, the path in longitudinal direction of a water parcel then follows a random walk. This condition only holds for estuaries of sufficient length; it may not be the case for wide, multichannel estuaries. The longitudinal displacements <math>X (1), X (2), … </math> in successive time intervals <math>\Delta T</math> are uncorrelated, by choosing <math>\Delta T=nT</math> equal to an integer number of cyclic tidal periods such that <math>\Delta T \ge T_A</math>. After a time <math>\tau</math> comprising a large number <math>N</math> of time intervals <math>\Delta T</math> the average net displacement of all water parcels initially situated in a given cross-section of the tidal basin is approximately zero in the absence of river discharge: | |
<math>E[\sum_{i=0}^N X (i)] \; \approx \; 0 , \qquad(1)</math> | <math>E[\sum_{i=0}^N X (i)] \; \approx \; 0 , \qquad(1)</math> | ||
| Line 19: | Line 26: | ||
where <math>E[..]</math> is the ensemble average over all water parcels initially situated in a given cross-section of the tidal basin. Here we have assumed <math>\tau \le T_x</math>. Because <math>X(i)</math> and <math>X(j)</math> are uncorrelated for <math>i \ne j</math>, we have <math>E[X(i)X(j)] \approx 0 \;</math> for <math>i \ne j</math> and <math>E[X(i)^2] \approx \overline{X^2} \;</math> is the average square displacement over a time <math>\Delta T</math>. The average square displacement over the time interval <math>\tau =N \Delta T</math> is therefore given by | where <math>E[..]</math> is the ensemble average over all water parcels initially situated in a given cross-section of the tidal basin. Here we have assumed <math>\tau \le T_x</math>. Because <math>X(i)</math> and <math>X(j)</math> are uncorrelated for <math>i \ne j</math>, we have <math>E[X(i)X(j)] \approx 0 \;</math> for <math>i \ne j</math> and <math>E[X(i)^2] \approx \overline{X^2} \;</math> is the average square displacement over a time <math>\Delta T</math>. The average square displacement over the time interval <math>\tau =N \Delta T</math> is therefore given by | ||
| − | <math>E[(\sum_{i=0}^N X (i))^2] \; = \; E[\sum_{i=0}^N X (i) \; \sum_{j=0}^N X (j)] \; \approx \; \sum_{i=0}^N E[X (i)^2] \; \approx \; \tau \; \overline{X^2} / \Delta T \qquad(2)</math> | + | <math>E[(\sum_{i=0}^N X (i))^2] \; = \; E[\sum_{i=0}^N X (i) \; \sum_{j=0}^N X (j)] \; \approx \; \sum_{i=0}^N E[X (i)^2] \; \approx \; \tau \; \overline{X^2} / \Delta T . \qquad(2)</math> |
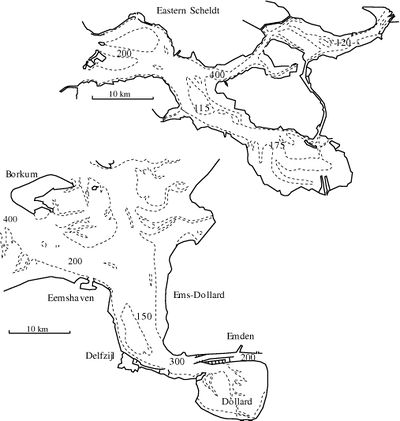
[[Image:OSEDdispersion.JPG|thumb|400px|right|Figure 1: Longitudinal dispersion coefficients at different locations in the Eastern Scheldt and Ems-Dollard estuaries.]] | [[Image:OSEDdispersion.JPG|thumb|400px|right|Figure 1: Longitudinal dispersion coefficients at different locations in the Eastern Scheldt and Ems-Dollard estuaries.]] | ||
| Line 25: | Line 32: | ||
The square of the average displacement of water parcels in the tidal basin thus increases linearly with time. This is precisely the characteristic of a diffusion process of a dissolved substance with concentration <math>s(x,t)</math>. This diffusion process is described by the equation<ref> Taylor, G.I., 1921, Diffusion by Continuous Movements. Proc., London Math. Soc., Ser. A 20: 196-211 </ref> | The square of the average displacement of water parcels in the tidal basin thus increases linearly with time. This is precisely the characteristic of a diffusion process of a dissolved substance with concentration <math>s(x,t)</math>. This diffusion process is described by the equation<ref> Taylor, G.I., 1921, Diffusion by Continuous Movements. Proc., London Math. Soc., Ser. A 20: 196-211 </ref> | ||
| − | <math>\Large \frac{\partial s}{\partial t} \normalsize = K_x \Large \frac{\partial^2 s}{\partial x^2} \normalsize , \quad </math> with diffusion coefficient <math>\quad K_x = \Large \frac{\overline{X^2} }{ 2 \Delta T} \normalsize \qquad(3)</math> | + | <math>\Large \frac{\partial s}{\partial t} \normalsize = K_x \Large \frac{\partial^2 s}{\partial x^2} \normalsize , \quad </math> with diffusion coefficient <math>\quad K_x = \Large \frac{\overline{X^2} }{ 2 \Delta T} \normalsize . \qquad(3)</math> |
The random walk assumption <math> T_x >> T_A</math>, expressing the condition that particle paths become uncorrelated for time intervals larger than the cross-sectional mixing time <math>T_A</math> but much smaller than the longitudinal mixing time <math>T_x</math>, thus implies that salt transport by seawater intrusion processes, <math>Q_{disp}</math>, can be represented by a gradient-type transport formula, | The random walk assumption <math> T_x >> T_A</math>, expressing the condition that particle paths become uncorrelated for time intervals larger than the cross-sectional mixing time <math>T_A</math> but much smaller than the longitudinal mixing time <math>T_x</math>, thus implies that salt transport by seawater intrusion processes, <math>Q_{disp}</math>, can be represented by a gradient-type transport formula, | ||
| Line 31: | Line 38: | ||
<math>Q_{disp} = - A_0 K_x \Large \frac{ds_0}{dx} \normalsize , \qquad(4) </math> | <math>Q_{disp} = - A_0 K_x \Large \frac{ds_0}{dx} \normalsize , \qquad(4) </math> | ||
| − | where <math>s_0</math> is the tidally averaged salinity concentration, <math>K_x </math> is the longitudinal dispersion coefficient and <math>A_0=<A></math>. | + | where <math>s_0</math> is the tidally averaged salinity concentration, <math>s_0(x)=<s(x,t)></math>, <math>K_x </math> is the longitudinal dispersion coefficient and <math>A_0=<A></math> (the brackets <> represent the average over a tidal cycle). |
The dispersion coefficient <math>K_x </math> has the important property that it does not depend explicitly on the salinity distribution in the estuary <ref>Dronkers, J. 1982. Conditions for gradient-type dispersive transport in one-dimensional tidally averaged transport models. Est.Coast.Shelf Sci. 14: 599-621</ref>, but only on the flow characteristics during the tidal cycle (which may be influenced by the salinity distribution, by the way). | The dispersion coefficient <math>K_x </math> has the important property that it does not depend explicitly on the salinity distribution in the estuary <ref>Dronkers, J. 1982. Conditions for gradient-type dispersive transport in one-dimensional tidally averaged transport models. Est.Coast.Shelf Sci. 14: 599-621</ref>, but only on the flow characteristics during the tidal cycle (which may be influenced by the salinity distribution, by the way). | ||
| − | The magnitude of the random displacements depends on the location <math>x </math> in the estuary; the longitudinal dispersion coefficient <math>K_x</math> is thus a function of <math>x </math>. This is illustrated in | + | The magnitude of the random displacements depends on the location <math>x </math> in the estuary; the longitudinal dispersion coefficient <math>K_x</math> is thus a function of <math>x </math>. This is illustrated in Fig. 1 for the Eastern Scheldt and Ems-Dollard estuaries. |
| Line 62: | Line 69: | ||
* Lateral mixing of water masses captured in "dead zones" with the main flow; | * Lateral mixing of water masses captured in "dead zones" with the main flow; | ||
* Chaotic dispersion, related to the chaotic character of particle trajectories when travelling through a complex field of tide-generated eddies; | * Chaotic dispersion, related to the chaotic character of particle trajectories when travelling through a complex field of tide-generated eddies; | ||
| − | * Tidal pumping at the inlet | + | * Tidal pumping at the inlet, which is defined here as the partial replacement of the ebb tidal prism with ‘new’ seawater flowing in from the nearshore zone during flood. |
| Line 69: | Line 76: | ||
These assumptions are: | These assumptions are: | ||
* the estuarine geometry does not vary strongly in <math>x</math>-direction over distances comparable to or larger than the tidal excursion; | * the estuarine geometry does not vary strongly in <math>x</math>-direction over distances comparable to or larger than the tidal excursion; | ||
| − | * the cross-section of the estuarine main channel | + | * the cross-section of the estuarine main channel can be represented approximately a rectangular shape. |
| − | We also have the condition <math>T_A<<T_x</math>. | + | We also have the condition <math>T_A<<T_x</math>, cross-sectional mixing takes place on a time scale much shorter than the estuarine flushing time. |
In the following we consider different seawater intrusion processes under these conditions and present an approximate analytical expression for the dispersive transport produced by each process. | In the following we consider different seawater intrusion processes under these conditions and present an approximate analytical expression for the dispersive transport produced by each process. | ||
| Line 80: | Line 87: | ||
<math>u_0 (z)= <u>-<\overline{u}^z> , \qquad(8) </math> | <math>u_0 (z)= <u>-<\overline{u}^z> , \qquad(8) </math> | ||
| − | where the brackets <math><u></math> stand for averaging over the tidal period | + | where the brackets <math><u></math> stand for averaging over the tidal period and <math>\overline{u}^z </math> for averaging over the vertical. The averaging is done in a frame moving with the cross-sectional mean velocity. The longitudinal dispersive transport can be estimated by a procedure outlined by G.I.Taylor <ref>Taylor, G.I. 1954. The dispersion of matter in turbulent flow through a pipe. Procs. Royal Society Londin A223: 446-468</ref>. The result is |
<math>K_x = f_0^{(z)} T_z \overline{(u_0)^2}^z , \qquad(9) </math> | <math>K_x = f_0^{(z)} T_z \overline{(u_0)^2}^z , \qquad(9) </math> | ||
| − | with <math> f_0^{(z)} </math> a coefficient of the order of 0.1 <ref>Fischer, H.B., List, E.J., Koh, R.C.Y., Imberger, J. and Brooks, N.H. 1979. Mixing in Inland and Coastal Waters. Academic Press, New York</ref>. | + | with <math> f_0^{(z)} </math> a coefficient of the order of 0.1 <ref>Fischer, H.B., List, E.J., Koh, R.C.Y., Imberger, J. and Brooks, N.H. 1979. Mixing in Inland and Coastal Waters. Academic Press, New York</ref>. The formula (9) shows that the dispersion coefficient increases with increasing mixing time <math>T_z</math>. However, there is a limit, because of the assumption <math>T_z << T_x</math>. |
| − | For the dispersion coefficient related to lateral horizontal residual circulation a similar formula can be derived, replacing in the expression for <math>K_x </math> everywhere <math>z</math> by <math>y</math>. | + | For the dispersion coefficient related to lateral horizontal residual circulation a similar formula can be derived, replacing in the expression for <math>K_x </math> everywhere <math>z</math> by <math>y</math>. Expressions for the vertical mixing time <math>T_z</math> and the lateral mixing time <math>T_y</math> are given in the section [[#Time scales for vertical and lateral mixing]]. |
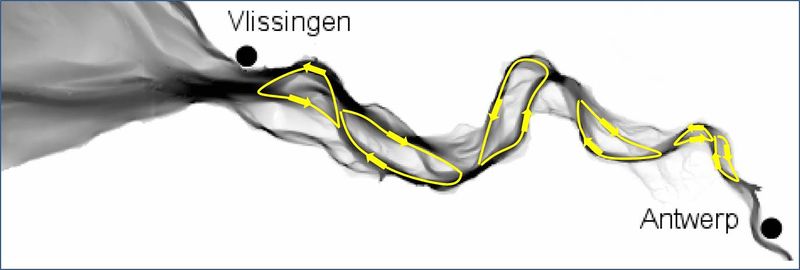
| − | Estuarine circulation is an important seawater intrusion mechanism in estuaries with a single deep (dredged) channel and small to moderate tide. Lateral circulations are important in wide natural estuaries with a complex geometry (meandering main channel , secondary channels, channel bars and tidal flats) and strong tides. The dominance of lateral circulations for seawater intrusion relative to vertical circulations appears in the analytical expression of <math>K_x </math> through the much larger lateral mixing time <math>T_y </math> compared to the vertical mixing time <math>T_z </math>. The presence of distinct ebb and flood channels is a major cause of lateral circulation in wide estuaries, see for example | + | Estuarine circulation is an important seawater intrusion mechanism in estuaries with a single deep (dredged) channel and small to moderate tide. Lateral circulations are important in wide natural estuaries with a complex geometry (meandering main channel , secondary channels, channel bars and tidal flats) and strong tides. The dominance of lateral circulations for seawater intrusion relative to vertical circulations appears in the analytical expression of <math>K_x </math> through the much larger lateral mixing time <math>T_y </math> compared to the vertical mixing time <math>T_z </math>. The presence of distinct ebb and flood channels is a major cause of lateral circulation in wide estuaries, see for example Fig. 2. However, density gradients related to seawater intrusion also produce lateral circulations (see [[Estuarine circulation]]), which contribute often even more to longitudinal dispersion than the vertical density-induced circulation <ref>Smith, R. 1980. Buoyance effects upon longitudinal dispersion in wide well-mixed estuaries. Philos. Trans. Royal Soc. London A 296: 467-496</ref>. |
| Line 95: | Line 102: | ||
==Dispersion by tidal straining== | ==Dispersion by tidal straining== | ||
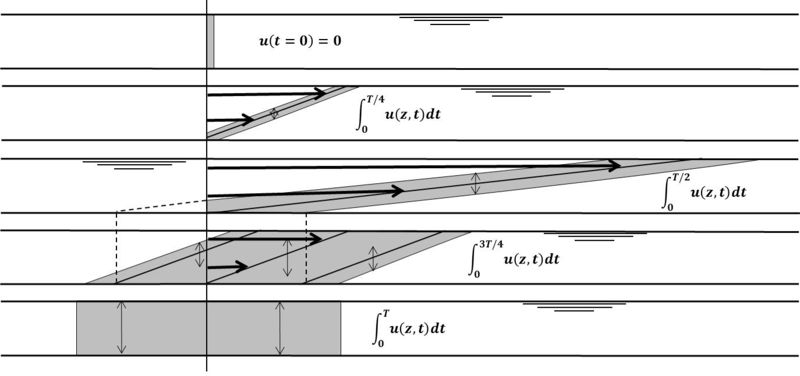
| − | If residual circulations are weak, dispersion is mainly caused by tidal straining, the relative displacement of water masses due to vertical and horizontal gradients in the tidal current velocity. In river flow, the usual term for this mixing mechanism is shear dispersion. Seawater intrusion in narrow deep estuaries is primarily caused by vertical tidal velocity gradients, whereas lateral tidal velocity gradients are important in wide estuaries. We present formulas for vertical tidal straining; the expressions for lateral tidal straining are similar. The process of longitudinal dispersion through tidal straining is explained in | + | If residual circulations are weak, dispersion is mainly caused by tidal straining, the relative displacement of water masses due to vertical and horizontal gradients in the tidal current velocity. In river flow, the usual term for this mixing mechanism is shear dispersion. Seawater intrusion in narrow deep estuaries is primarily caused by vertical tidal velocity gradients, whereas lateral tidal velocity gradients are important in wide estuaries. We present formulas for vertical tidal straining; the expressions for lateral tidal straining are similar. The process of longitudinal dispersion through tidal straining is explained in Fig. 3. |
The velocity component <math>u_1 (z,t) </math> responsible for vertical tidal straining is | The velocity component <math>u_1 (z,t) </math> responsible for vertical tidal straining is | ||
| Line 104: | Line 111: | ||
By a procedure outlined by Holley, Harleman and Fischer <ref>Holley, E.R., Harleman, D.R. and Fischer, H.B. 1970. Dispersion in homogeneous estuary flow. J. Hydr. Div. ASCE 96: 1691-1706</ref>, the following first-order estimate of the longitudinal dispersion coefficient is obtained: | By a procedure outlined by Holley, Harleman and Fischer <ref>Holley, E.R., Harleman, D.R. and Fischer, H.B. 1970. Dispersion in homogeneous estuary flow. J. Hydr. Div. ASCE 96: 1691-1706</ref>, the following first-order estimate of the longitudinal dispersion coefficient is obtained: | ||
| − | <math>K_x \approx f_1^{(z)} \Large \frac{T_z <\overline{ u_1^2}^z> }{1+( f_2^{(z)} T_z / T)^2 } \normalsize \qquad(11) </math> | + | <math>K_x \approx f_1^{(z)} \Large \frac{T_z <\overline{ u_1^2}^z> }{1+( f_2^{(z)} T_z / T)^2 } \normalsize . \qquad(11) </math> |
| − | The coefficients <math> f_1^{(z)}, f_2^{(z)} </math> depend on the velocity profile; order-of-magnitude estimates are <math> f_1^{(z)} \sim 0.1-0.2</math> and <math> f_2^{(z)} \sim 0.5-1 </math>. | + | The coefficients <math> f_1^{(z)}, f_2^{(z)} </math> depend on the velocity profile; order-of-magnitude estimates are <math> f_1^{(z)} \sim 0.1-0.2</math> and <math> f_2^{(z)} \sim 0.5-1 </math>. <math>T</math> is the tidal period. |
Longitudinal dispersion produced by lateral tidal straining can be expressed by a formula similar as for vertical tidal straining. Dispersion by tidal straining is largest if the time scale for vertical or lateral mixing is on the order of <math>T/ f_2</math>. The time scale for vertical mixing is generally smaller than the tidal period and the time scale for lateral mixing is generally larger. Dye experiments illustrating dispersion by lateral tidal straining are shown in [[Estuarine dispersion: dye experiments in the Eastern Scheldt scale model]]. | Longitudinal dispersion produced by lateral tidal straining can be expressed by a formula similar as for vertical tidal straining. Dispersion by tidal straining is largest if the time scale for vertical or lateral mixing is on the order of <math>T/ f_2</math>. The time scale for vertical mixing is generally smaller than the tidal period and the time scale for lateral mixing is generally larger. Dye experiments illustrating dispersion by lateral tidal straining are shown in [[Estuarine dispersion: dye experiments in the Eastern Scheldt scale model]]. | ||
| Line 113: | Line 120: | ||
| − | [[Image:DispersionTidalShear.JPG|thumb|800px|center|Figure 3: Schematic representation of longitudinal dispersion by a vertical gradient in the tidal current <math>u(z,t)</math>. A patch of dye is introduced homogeneously over the vertical when flood starts at low water slack tide (<math>t=0</math>). The patch of dye is stretched by the tidal current while being mixed vertically by turbulent diffusion. The time scale for vertical mixing <math>T_z</math> is assumed | + | [[Image:DispersionTidalShear.JPG|thumb|800px|center|Figure 3: Schematic representation of longitudinal dispersion by a vertical gradient in the tidal current <math>u(z,t)</math>. A patch of dye is introduced homogeneously over the vertical when flood starts at [[Slack water|low-water slack tide]] (<math>t=0</math>). The patch of dye is stretched by the tidal current while being mixed vertically by turbulent diffusion. The time scale for vertical mixing <math>T_z</math> is assumed of the same order as the tidal period <math>T</math>. The tidal cycle is represented by four discrete time steps, illustrating the combined action of turbulent vertical mixing and shear of the current in the vertical. The stretching of the dye patch combined with vertical diffusion results in a strong longitudinal spreading after a tidal cycle.]] |
| + | |||
| + | |||
| + | |||
| + | ==Time scales for vertical and lateral mixing== | ||
| + | A difficulty for practical use of the previous expressions for longitudinal dispersion, results from the uncertainty related to estimating the vertical and (especially) lateral mixing times, <math>T_z=D^2/K_z</math> and <math>T_y=B^2/K_y</math>. In case of a logarithmic velocity profile (in the absence of density stratification), the vertical diffusion coefficient is given by <math>K_z=0.4z(1-z/D)u_*</math>, where <math>u_*\approx 0.05 U</math> is the friction velocity and <math>U</math> the flow velocity. This yields a longitudinal dispersion coefficient <math>K_x \approx 6u_*D</math>, for steady flow <ref>Elder, J.W. 1959. The Dispersion of Marked Fluid in Turbulent Shear Flow. J. Fluid Mech. 5: 544-560 </ref>. However, in case of buoyant flows, vertical diffusion can be much slower (smaller <math>K_z</math>), leading to stronger longitudinal dispersion. | ||
| + | Lateral diffusion depends strongly on the geometry of the estuary. The lateral diffusion coefficient is generally expressed as <math>K_y \approx \alpha u_* D</math>. An empirical estimate for moderately meandering channels is <math>\alpha \approx 0.6 </math> <ref>Fischer H.B. 1972. Mass transport mechanisms in partially stratified estuaries. J. Fluid Mech. 53: 671–687 </ref> and a model estimate is <math> \alpha \approx 150 (B/ R)^2 </math> <ref>Yotsukura, N. and Sayre, W.W. 1976. Transverse mixing in natural channels. Water Resources Res. 12: 695–704</ref>, where <math>B</math> is the channel width and <math>R</math> is a characteristic channel bend radius. | ||
==Dispersion by “dead zones”== | ==Dispersion by “dead zones”== | ||
| − | The formula for lateral tidal straining | + | The formula for lateral tidal straining may include the influence of "dead zones", if they are considered part of the estuarine cross-section and if they are distributed regularly along the estuary. Dead zones are areas along the main estuarine channel where water is stored during high water, without being significantly transported in longitudinal direction, for instance, tidal flats or lateral creeks (these dead zones should not be confused with the anoxic zones, called 'dead zones' in ecology). The longitudinal dispersion coefficient is given by an expression of the type <ref>Okubo, A. 1967. The effect of shoreline irregularities on horizontal diffusion from an instantaneous source. Inter. J. Oceanol. Limnol. 1: 194-204</ref> |
| + | |||
| + | <math>K_x=f_{dz} \, r \, U^2T , \qquad(12) </math> | ||
| − | <math> | + | where <math>U</math> is the maximum tidal velocity, <math>T</math> the tidal period and <math>r</math> is the high-water (HW) storage cross-section of the dead zones relative to the channel cross-section. The coefficient <math>f_{dz}</math> depends on the mixing rate within the dead zone; in case of complete mixing during the tidal cycle <math>f_{dz}=1/12 \pi^2</math>, assuming that filling of the dead zones starts at low water (LW) <ref name=”Dron”>Dronkers, J. 1978. Longitudinal dispersion in shallow well-mixed estuaries. Procs. 16th Int. Conf. Coastal Eng. 3: 2761-2777</ref>. |
| − | + | Even without any mixing, storage areas along the channel contribute to longitudinal dispersion, because of a non-zero phase shift <math>\phi</math> that generally exists between horizontal and vertical tidal motion (i.e., phase shift between <math>u(t)</math> and <math>d\eta /dt</math>, respectively, where <math>\eta(t)</math> is the tidal level). We assume dead zones with bed level at low water (or below), which are distributed evenly along the estuarine main channel. Fluid parcels that are stored temporarily in the dead zone experience a net seaward displacement <math>\Delta X</math> relative to fluid parcels that remain in the channel, see Fig. 4. Filling and emptying of the dead zones during the tidal period then produces a net transport through a plane at <math>x=0</math> given by | |
| − | + | <math>Q_{disp} = \Large \frac{1}{T} \large \int_0^{T/2} \Large \frac{d A_s}{dt} \normalsize dt \large \int_0^{|\Delta X(t)|} \normalsize [s(x,0)-s(0,0)] dx , \qquad(13) </math> | |
| − | <math> | + | where <math>A_s(t) = b_s \eta (t) </math> is the dead zone volume at time <math>t</math> per unit estuarine length, <math> s(x,t)-s(0,t) \approx x \, ds_0/dx</math> and <math>\Delta X(t)= -(2U/\omega) \sin \phi \sin \omega t</math>. The integral evaluates mass transport due to water parcels passing through the plane <math>x=0</math> during ebb but not during flood (represented by the first term <math>s(x,t)</math> between the square brackets), taking into account an equivalent landward water flow in the channel (second term <math>s(0,t)</math> between the square brackets). By evaluating the integral we find for the coefficient <math>f_{dz}</math> the expression |
| − | + | <math>f_{dz} = \Large \frac{\sin^2 \phi}{3 \pi^2} \normalsize . \qquad (14) </math> | |
A usual order of magnitude for the phase shift <math>T\phi/2 \pi</math> is 30-60 minutes, yielding <math>f_{dz} \approx 0.005 </math>. In estuaries with large tidal flats dead zones can significantly enhance longitudinal dispersion. | A usual order of magnitude for the phase shift <math>T\phi/2 \pi</math> is 30-60 minutes, yielding <math>f_{dz} \approx 0.005 </math>. In estuaries with large tidal flats dead zones can significantly enhance longitudinal dispersion. | ||
| Line 134: | Line 149: | ||
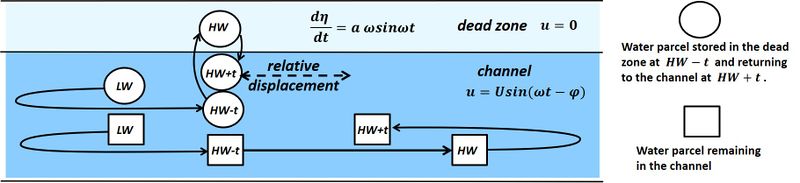
| − | [[Image:DeadZoneDispersion.JPG|thumb|800px|center|Figure 4: Dispersion by an intertidal "dead zone" along a tidal channel. The figure illustrates the case of a phase shift <math>\phi</math> between the vertical tidal motion (<math>d\eta /dt</math>) and the horizontal tidal motion (<math>u(t)</math>). The paths of two fluid parcels are shown, both starting from the same location at low water (<math>t=0</math>). The first parcel (the square) follows the tidal motion in the channel, while the second parcel (the circle) is stored in the dead zone around high water in a time interval <math>[t, T | + | [[Image:DeadZoneDispersion.JPG|thumb|800px|center|Figure 4: Dispersion by an intertidal "dead zone" along a tidal channel. The figure illustrates the case of a phase shift <math>\small \phi</math> between the vertical tidal motion (<math>\small d\eta /dt</math>) and the horizontal tidal motion (<math>\small u(t)</math>). The paths of two fluid parcels are shown, both starting from the same location at low water (<math>\small t=0</math>). The first parcel (the square) follows the tidal motion in the channel, while the second parcel (the circle) is stored in the dead zone around high water in a time interval <math>\small [T/2-t, T/2+t]</math>. Without mixing, the latter parcel returns to the channel at time <math>\small T/2+t</math> at the same position where it entered the death zone, <math>\small X_1(t)=(U/\omega)[\cos \phi - \cos(\pi - \omega t - \phi)]</math>. This is seaward from the position <math>\small X_2(t)=(U/\omega)[\cos \phi - \cos(\pi + \omega t - \phi)]</math> at that time of the parcel that remained in the channel. The relative seaward displacement of particles leaving the dead zone at time <math>\small T/2-t</math> is given by <math>\small \Delta X(t)=-(2U / \omega) \sin \phi \sin \omega t .</math> The result is longitudinal dispersion: a net relative displacement of the two fluid parcels during the tidal cycle. ]] |
| + | |||
| + | In the same way it is possible to estimate the influence of a small lateral creek on the longitudinal dispersion in the estuary. Assuming that filling and emptying of the creek is in phase with the vertical tidal motion <math>d \eta /dt</math>, that the phase difference <math>\phi</math> with the horizontal tidal motion <math>u(x,t)</math> is small and that no mixing takes place, the contribution of the tidal creek to the longitudinal dispersion in the estuary is given by <ref name=”Dron”></ref> | ||
| − | = | + | <math>K_x = \sin^2 \phi \, \Large\frac{VL}{2A_0} (1 – (\frac{2x}{L} )^2)\normalsize , \qquad (15) </math> |
| − | + | ||
| − | + | where <math>V</math> is the tidal storage volume of the creek, <math>A_0</math> is the mean channel cross-section, <math>L=UT/\pi</math> is the tidal excursion and <math>x</math> is the along-channel distance to the creek entrance. Equation (15) illustrates why the longitudinal dispersion coefficient can be variable along the estuary. Integration of (15) along the estuary yields the expression (14). | |
==Dispersion by deterministic chaos== | ==Dispersion by deterministic chaos== | ||
| − | |||
| − | <math>K_x \propto UL \qquad( | + | [[Image:ChaoticDispersion.jpg|thumb|300px|left|Figure 5: Stretching of an initially rectangular patch of dye in a few tidal cycles, according to a 2D numerical hydrodynamic model of the central part of the Western Wadden Sea <ref>Ridderinkhof, H., Zimmerman, J. T. F. 1990. Mixing Processes in a Numerical Model of the Western Dutch Wadden Sea. In: Coastal and Estuarine Studies, Vol. 38 Residual Currents and Long·term Transport, R. T. Cheng (Ed.), Springer</ref>.]] |
| + | |||
| + | Dye experiments show that dispersion in wide estuaries with complex geometry generally proceeds in an irregular way, by advection of fluid parcels through a field of geometry-induced tidal eddies. The result is very different from turbulent diffusion (i.e. diffusion by a cascade of turbulent eddies of progressively decreasing size). Parts of the dye can be trapped within gyres without any significant turbulent diffusion, while other dye patches can be highly stretched in the flow direction. Strong stretching occurs in particular in the interface zones between tidal eddies, see Fig. 5. Zimmerman (1986) <ref name=Z> Zimmerman, J.T.F. 1986. The Tidal Whirlpool: A Review of Horizontal Dispersion by Tidal and Residual Currents. Neth. Journal of Sea Research 20:133-154 </ref> described the dispersion process in such systems as the result of Lagrangian chaos produced by 'a tidal whirlpool'. Fluid parcels can be dispersed over the entire length of the estuary before lateral mixing has taken place. In this case, the random walk description of tidal dispersion is no longer valid. Zimmerman showed that longitudinal dispersion can still be described as a random process, even if turbulent mixing is completely absent. He called this random process “deterministic chaos” <ref>Ridderinkhof, H. and Zimmerman, J. T. F. 1992. Chaotic stirring in a tidal system. Science 258: 1107-1109</ref>. In his model, fluid parcels are dispersed by moving along chaotic orbits through a lattice of tidal eddies. Most dispersion is generated by eddies with a size <math>\lambda</math> comparable to the tidal excursion length <math>L</math> <ref> Zimmerman, J.T.F. 1976. Mixing and flushing of tidal embayments in the western Wadden Sea, I: Distribution of salinity and calculation of mixing time scales. Neth.J.Sea Res. 10:149-191 </ref>. This suggests that the longitudinal dispersion coefficient for chaotic mixing should be proportional to | ||
| + | |||
| + | <math>K_x \propto UL . \qquad(16) </math>. | ||
The size of the eddies also depends on the basin with <math>B</math>. A field study in Willapa Bay (US Pacific coast) suggests that chaotic dispersion could be described by a dispersion coefficient <math>K_x = 0.06 UB</math> <ref>Banas, N.S., Hickey, B.M., 2005. Mapping exchange and residence time in a model of Willapa Bay, Washington, a branching, macrotidal estuary. Journal of Geophysical Research 110, C11011. doi:10.1029/2005JC002950</ref>. If the lateral mixing time <math>T_y</math> is comparable to or larger than the flushing time <math>T_x </math>, the representation of the dispersive transport <math> Q_{disp}</math> by the product of a dispersion coefficient and the local salinity gradient is no longer valid. | The size of the eddies also depends on the basin with <math>B</math>. A field study in Willapa Bay (US Pacific coast) suggests that chaotic dispersion could be described by a dispersion coefficient <math>K_x = 0.06 UB</math> <ref>Banas, N.S., Hickey, B.M., 2005. Mapping exchange and residence time in a model of Willapa Bay, Washington, a branching, macrotidal estuary. Journal of Geophysical Research 110, C11011. doi:10.1029/2005JC002950</ref>. If the lateral mixing time <math>T_y</math> is comparable to or larger than the flushing time <math>T_x </math>, the representation of the dispersive transport <math> Q_{disp}</math> by the product of a dispersion coefficient and the local salinity gradient is no longer valid. | ||
| + | |||
| + | |||
| + | |||
| + | |||
==Dispersion by tidal pumping== | ==Dispersion by tidal pumping== | ||
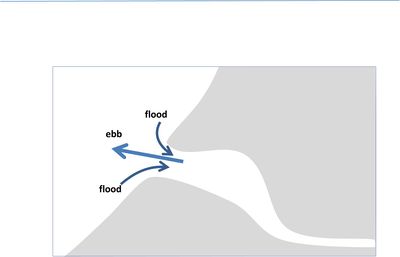
| − | Salt intrusion by tidal pumping is related to the higher average salinity of seawater entering the estuary during flood compared to the average salinity of estuarine water flowing out into the sea during ebb. It depends on the rate of renewal of estuarine water in the outflow region and therefore on tidal inflow-outflow hydrodynamics. Outflow of estuarine water often has a jet-like character, whereas flood water enters the estuary more distributed from different directions, depending on the tidal phase, see | + | Salt intrusion by tidal pumping is related to the higher average salinity of seawater entering the estuary during flood compared to the average salinity of estuarine water flowing out into the sea during ebb. It depends on the rate of renewal of estuarine water in the outflow region and therefore on tidal inflow-outflow hydrodynamics. Outflow of estuarine water often has a jet-like character, whereas flood water enters the estuary more distributed from different directions, depending on the tidal phase, see Fig. 6. The inflowing flood water therefore contains ‘new’ seawater from the sides of the ebb channel. Replacement of outflowing estuarine water by "new" seawater is enhanced when river discharge is high, because the ebb jet is concentrated in a surface layer (salinity stratification), whereas seawater inflow is more evenly distributed over the vertical. |
| − | [[Image:TidalPumping.JPG|thumb|400px|right|Figure | + | [[Image:TidalPumping.JPG|thumb|400px|right|Figure 6: Schematic representation of seawater intrusing by tidal pumping.]] |
We call <math>\alpha</math> the replacement rate of outflowing estuarine water by seawater during a tidal cycle. For small fresh water discharge, the corresponding dispersion coefficient at the estuarine mouth is given by | We call <math>\alpha</math> the replacement rate of outflowing estuarine water by seawater during a tidal cycle. For small fresh water discharge, the corresponding dispersion coefficient at the estuarine mouth is given by | ||
| − | <math>K_{mouth} = \alpha \Large \frac{L^2 }{2T} , \normalsize \qquad( | + | <math>K_{mouth} = \alpha \Large \frac{L^2 }{2T} , \normalsize \qquad(17) </math> |
where <math>L=\int_{flood} u(t)dt </math> is the tidal excursion at the estuarine mouth. | where <math>L=\int_{flood} u(t)dt </math> is the tidal excursion at the estuarine mouth. | ||
| − | Savenije <ref name=”Sav”> Savenije, H. H. G. 2005. Salinity and Tides in Alluvial Estuaries. Elsevier, Amsterdam, 197 pp.</ref> derived the following empirical expression for <math>\alpha</math>: | + | Savenije <ref name=”Sav”> Savenije, H. H. G. 2005. Salinity and Tides in Alluvial Estuaries. Elsevier, Amsterdam, 197 pp.</ref> derived the following empirical expression for <math>\alpha</math>, based on observations suggesting that the renewal of estuarine water in the mouth area depends on the buoyancy of the ebb jet: |
| − | <math>\alpha=\Large \frac{2800 \pi D}{l_A} \normalsize \sqrt{Ri_E} | + | <math>\alpha=\Large \frac{2800 \pi D}{l_A} \normalsize \sqrt{Ri_E} . \qquad(18) </math> |
| − | + | In this expression <math>D</math> is the depth and <math>l_A</math> the convergence length of the estuary in the outflow zone (assuming exponential convergence of the cross-section in the outflow zone, <math> A(x) \sim exp(-x/l_A)</math>); | |
<math>Ri_E</math> is the Richardson estuary number <math>Ri_E=g \Delta \rho Q_R T^3 / ( \pi^2 \rho B L^3)</math>; | <math>Ri_E</math> is the Richardson estuary number <math>Ri_E=g \Delta \rho Q_R T^3 / ( \pi^2 \rho B L^3)</math>; | ||
| Line 175: | Line 199: | ||
The residence time <math>T_r</math> is defined as the average time a water parcel, which is initially located at a distance <math>x</math> from the sea boundary, will take to leave the estuary. If the fresh water discharge is zero or very small, and if the dispersion coefficient <math>K_x</math> is assumed constant along the estuary, the residence time is given by random walk theory <ref>Zimmerman, J.T.F. 1981 The flushing of well mixed tidal lagoons and its seasonal fluctuations. UNESCO Tech. Pap. Mar. Sci. 33: 15-26 </ref>: | The residence time <math>T_r</math> is defined as the average time a water parcel, which is initially located at a distance <math>x</math> from the sea boundary, will take to leave the estuary. If the fresh water discharge is zero or very small, and if the dispersion coefficient <math>K_x</math> is assumed constant along the estuary, the residence time is given by random walk theory <ref>Zimmerman, J.T.F. 1981 The flushing of well mixed tidal lagoons and its seasonal fluctuations. UNESCO Tech. Pap. Mar. Sci. 33: 15-26 </ref>: | ||
| − | <math>T_r = \Large \frac{x^2}{2K_x} \normalsize \qquad( | + | <math>T_r = \Large \frac{x^2}{2K_x} \normalsize . \qquad(19) </math> |
The flushing time <math>T_f=T_x</math> is the average time it takes for a fresh water parcel to move through the estuarine zone to the sea. According to the random walk model, for small discharge <math>Q_R</math>, | The flushing time <math>T_f=T_x</math> is the average time it takes for a fresh water parcel to move through the estuarine zone to the sea. According to the random walk model, for small discharge <math>Q_R</math>, | ||
| − | <math>T_f = \Large \frac{l^2}{2K_x} , \normalsize \qquad( | + | <math>T_f = \Large \frac{l^2}{2K_x} , \normalsize \qquad(20) </math> |
where <math>l</math> is the estuarine length. This is approximately equivalent to | where <math>l</math> is the estuarine length. This is approximately equivalent to | ||
| Line 187: | Line 211: | ||
==Experimental determination of the longitudinal dispersion coefficient== | ==Experimental determination of the longitudinal dispersion coefficient== | ||
| − | The dispersion coefficient <math>K_x</math> can be determined experimentally in situations where the freshwater discharge <math>Q_R</math> is constant over a period longer than <math>T_x</math>. In that case the salinity distribution <math>s(x,t)</math> is close to equilibrium (<math> s(x,t)\approx s_0(x) </math>). The total residual salt flux <math>Q_S</math> approximately equals zero. We thus have (with the sign convention <math>Q_R > 0</math>) | + | The dispersion coefficient <math>K_x</math> can be determined experimentally in situations where the freshwater discharge <math>Q_R</math> is constant over a period longer than <math>T_x</math>. In that case the salinity distribution <math><s(x,t)></math> is close to equilibrium (<math> <s(x,t)>\approx s_0(x) </math>). The total residual salt flux <math>Q_S</math> approximately equals zero. We thus have (with the sign convention <math>Q_R > 0</math>) |
| − | <math>AK_x \Large \frac{ds_0}{dx} \normalsize + Q_R s_0 = 0 \qquad( | + | <math>AK_x \Large \frac{ds_0}{dx} \normalsize + Q_R s_0 = 0 . \qquad(21) </math> |
| − | Values of the dispersion coefficient can be derived from | + | Values of the dispersion coefficient can be derived from measurements of the residual discharge <math>Q_R</math> and the salinity distribution <math>s_0(x)</math>. Estimates of longitudinal dispersion coefficients determined in this way are shown in Table 1. More detailed analyses show that the dispersion coefficient <math>K_x</math> is a function of <math>x</math> and <math>Q_R</math>. The dependence of <math>K_x</math> on <math>Q_R</math> has two causes. It is related in the first place to the influence of the salinity distribution on the velocity flow field <math>u(x,u,z,t)</math>; such an influence is due to salinity-induced density gradients (see [[Estuarine circulation]]). In the second place, it is related to the location of the freshwater-seawater transition zone. If this zone is situated near the estuarine mouth, the dispersion coefficient is strongly influenced by tidal pumping. This explains the high longitudinal dispersion coefficients for Rotterdam Waterway, Seine and Loire in Table 1, which are determined for situations with important river flow <math>Q_R/hb</math>. The same holds, to a somewhat lesser degree, for the Elbe, Weser, Mekong and Sinnamary. |
| − | + | The influence of salinity-induced density gradients on the longitudinal dispersion coefficient is generally small in estuaries with complex topography and small river inflow (<math>Q_R/hb</math> several orders of magnitude smaller than the tidal velocity). This is the case for many tidal lagoons, such as the Wadden Sea. | |
If a non-buoyant dissolved substance is introduced in the estuary, it will be mixed over the cross-section after a time <math>T_A</math> . From that time, the longitudinal dispersion of the substance is similar to salinity dispersion (same dispersion coefficient). For a permanent discharge of a non-buoyant dissolved substance, the same dispersion coefficient applies in the estuarine zones where the substance is mixed over the estuarine cross-section. | If a non-buoyant dissolved substance is introduced in the estuary, it will be mixed over the cross-section after a time <math>T_A</math> . From that time, the longitudinal dispersion of the substance is similar to salinity dispersion (same dispersion coefficient). For a permanent discharge of a non-buoyant dissolved substance, the same dispersion coefficient applies in the estuarine zones where the substance is mixed over the estuarine cross-section. | ||
| Line 452: | Line 476: | ||
|AuthorName=Dronkers J}} | |AuthorName=Dronkers J}} | ||
| + | [[Category:Physical coastal and marine processes]] | ||
[[Category:Estuaries and tidal rivers]] | [[Category:Estuaries and tidal rivers]] | ||
| − | [[Category:Hydrodynamics | + | [[Category:Hydrodynamics]] |
| − | |||
| − | |||
Latest revision as of 14:07, 2 July 2023
We describe in this article the physical processes involved in seawater intrusion and mixing in estuaries under the influence of tidal motion and explain some simple methods for deriving quantitative estimates. Several of the dispersion mechanisms discussed in this article are illustrated by dye experiments shown in Estuarine dispersion: dye experiments in the Eastern Scheldt scale model.
Contents
- 1 Introduction
- 2 Definitions and assumptions
- 3 Random walk
- 4 Longitudinal dispersion mechanisms
- 5 Analytical expressions for the longitudinal dispersion coefficient
- 6 Dispersion by residual circulation
- 7 Dispersion by tidal straining
- 8 Time scales for vertical and lateral mixing
- 9 Dispersion by “dead zones”
- 10 Dispersion by deterministic chaos
- 11 Dispersion by tidal pumping
- 12 Residence time scale
- 13 Experimental determination of the longitudinal dispersion coefficient
- 14 Related articles
- 15 References
Introduction
Estuaries are generally defined as semi-enclosed transition zones between river and sea. The intrusion of seawater in estuaries determines the salinity distribution. Salinity is usually expressed as the weight in grams of all dissolved salts per kilogram of water or alternatively according to the Practical Salinity Scale (see Salinity). The seawater intrusion limit is determined by the location in the estuary where the difference with river salinity is below a small number. Seawater intrusion is mainly due to tides and to the influence of the density difference between seawater and river water on currents and turbulence (this influence is often termed 'buoyancy effects', see the article Estuarine circulation for further details). It is counteracted by freshwater inflow from rivers, groundwater, and other sources. Seawater intrusion in estuaries is an important phenomenon to man and nature: it limits freshwater availability for human and agricultural use, and it determines the type of habitats and species that can develop in an estuarine environment. Besides, density driven currents and salinity play a role in estuarine turbidity and sedimentation processes.
The net effect of estuarine mixing processes during a tidal cycle on along-channel seawater intrusion or on net along-channel spreading (diffusion) of dissolved substances is generally called longitudinal dispersion. This net effect is described by a longitudinal dispersion coefficient [math]K_x[/math]. The different mixing processes contributing to longitudinal dispersion are termed dispersion mechanisms. In well-mixed and partially mixed estuaries these mixing processes are dominantly induced by tidal motion; the net effect on seawater intrusion is called tidal longitudinal dispersion or simply tidal dispersion. Longitudinal dispersion and the flushing time of an estuary are related concepts. The combined effect of all tidal dispersion processes on seawater intrusion is sometimes called 'tidal pumping'. Here we use the term 'tidal pumping' only for the fraction of outflowing estuarine water that is replaced by 'new' seawater at the estuarine mouth.
Seawater intrusion has a strong variability. At a fixed station, the salinity increases during high tide and is greatest at high water; the salinity decreases during low tide and is smallest at low tide. Besides, seawater intrusion is small with high river discharge and strong with low river discharge. Change in seawater intrusion with river discharge is more gradual than the variability due to flood and ebb. If the river discharge varies on a time scale greater than the flushing time of the estuary, the tide-averaged salinity distribution tends towards an equilibrium distribution. The longitudinal dispersion coefficient can be determined by measuring the salinity distribution under equilibrium conditions. This coefficient generally varies along the estuary and depends on the river discharge.
The dispersion processes that are responsible for seawater intrusion determine the flushing time as well as the residence time of dissolved substances in an estuary. This holds similarly for the residence time of micro-organisms that are carried along passively (not swimming, floating or settling) with the water.
Definitions and assumptions
The estuarine mouth is defined as the transition between zones of channeled tidal flow (tidal discharge mainly following the channel axis) and non-channeled flow (discharge deviated along the open coast). The estuarine head is defined here as the location where the seawater influence on the salinity is (almost) nil; this location depends on the river discharge. We are interested in the net effect of tidal mixing processes during a tidal cycle on seawater intrusion along the estuarine axis, defined as the [math]x[/math]-axis ([math]x=0[/math] at the estuarine mouth). This makes sense only if we assume that the salinity difference between the estuarine mouth and the estuarine head is much greater than salinity differences in lateral ([math]y[/math]-axis) and vertical ([math]z[/math]-axis) directions. Estuaries where salinity differences between bottom and surface are comparable to the salinity differences between estuarine mouth and estuarine head are dealt with in the article Salt wedge estuaries. The estuaries considered in this article are often designated as well-mixed or partially mixed estuaries.
Successive tidal cycles are not identical. Determining the net effect of tidally induced mixing processes requires integration over a large number of tidal cycles. To prevent this complication, we assume that successive tidal cycles are sufficiently comparable (no extreme spring-neap variation) to enable the definition of a representative cyclic tide. This assumption will be used throughout this article.
Random walk
Under certain conditions, estuarine mixing processes can be described in terms of random walk. For simplicity we consider a tidal basin with small river inflow (river discharge much smaller than tidal discharges). Situations where river flow exerts a substantial influence on estuarine water motion are dealt with in the article Estuarine circulation. Water motion is described as the average motion of a very large number of individual water parcels. If individual water parcels follow identical paths during flood (inflow) and ebb (outflow) all seawater parcels entering the basin during flood will be expelled during ebb: no seawater intrusion will occur. In reality this will not happen; mixing processes ensure that individual water parcels follow different paths during flood and ebb. Water parcels move back and forth in a tidal basin for some time before finally leaving the estuary and being evacuated offshore. We call [math] T_x [/math] the flushing time of water parcels in the tidal basin; it is defined as the average time it takes for a fluid parcel to travel from the upstream boundary of the estuary to the sea. During this time, water parcels also move in lateral and vertical directions, due to flow circulations and turbulent eddies. The time scale for vertical mixing, [math]T_z [/math], and the time scale for lateral mixing, [math]T_y [/math], are related to the vertical and lateral turbulent diffusion coefficients, [math]K_z [/math] and [math]K_y [/math], by the relationships [math]T_z=D^2/K_z [/math] and [math]T_y=B^2/K_y [/math], respectively. The time scales [math]T_y [/math] and [math]T_z [/math] indicate the time during which the motions of individual water parcels remain correlated; after a longer time they have lost the memory of their initial position [math](y_0,z_0)[/math] in the estuarine cross-section. The probability that after this time they will be in a cross-sectional position [math](y,z)[/math] from where their net displacement during the next tidal cycle will be in landward direction is then equal to the probability that they will be in a position where their net displacement will be in seaward direction, if the influence of river discharge is ignored. The cross-sectional mixing time [math]T_A [/math] is approximately equal to the lateral mixing time [math]T_y[/math], if we assume that lateral mixing takes more time than vertical mixing; this is the case for most well-mixed or partially mixed wide estuaries. If [math]T_A[/math] is much smaller than the longitudinal flushing time [math]T_x[/math] of water parcels in the estuary, the path in longitudinal direction of a water parcel then follows a random walk. This condition only holds for estuaries of sufficient length; it may not be the case for wide, multichannel estuaries. The longitudinal displacements [math]X (1), X (2), … [/math] in successive time intervals [math]\Delta T[/math] are uncorrelated, by choosing [math]\Delta T=nT[/math] equal to an integer number of cyclic tidal periods such that [math]\Delta T \ge T_A[/math]. After a time [math]\tau[/math] comprising a large number [math]N[/math] of time intervals [math]\Delta T[/math] the average net displacement of all water parcels initially situated in a given cross-section of the tidal basin is approximately zero in the absence of river discharge:
[math]E[\sum_{i=0}^N X (i)] \; \approx \; 0 , \qquad(1)[/math]
where [math]E[..][/math] is the ensemble average over all water parcels initially situated in a given cross-section of the tidal basin. Here we have assumed [math]\tau \le T_x[/math]. Because [math]X(i)[/math] and [math]X(j)[/math] are uncorrelated for [math]i \ne j[/math], we have [math]E[X(i)X(j)] \approx 0 \;[/math] for [math]i \ne j[/math] and [math]E[X(i)^2] \approx \overline{X^2} \;[/math] is the average square displacement over a time [math]\Delta T[/math]. The average square displacement over the time interval [math]\tau =N \Delta T[/math] is therefore given by
[math]E[(\sum_{i=0}^N X (i))^2] \; = \; E[\sum_{i=0}^N X (i) \; \sum_{j=0}^N X (j)] \; \approx \; \sum_{i=0}^N E[X (i)^2] \; \approx \; \tau \; \overline{X^2} / \Delta T . \qquad(2)[/math]
The square of the average displacement of water parcels in the tidal basin thus increases linearly with time. This is precisely the characteristic of a diffusion process of a dissolved substance with concentration [math]s(x,t)[/math]. This diffusion process is described by the equation[1]
[math]\Large \frac{\partial s}{\partial t} \normalsize = K_x \Large \frac{\partial^2 s}{\partial x^2} \normalsize , \quad [/math] with diffusion coefficient [math]\quad K_x = \Large \frac{\overline{X^2} }{ 2 \Delta T} \normalsize . \qquad(3)[/math]
The random walk assumption [math] T_x \gt \gt T_A[/math], expressing the condition that particle paths become uncorrelated for time intervals larger than the cross-sectional mixing time [math]T_A[/math] but much smaller than the longitudinal mixing time [math]T_x[/math], thus implies that salt transport by seawater intrusion processes, [math]Q_{disp}[/math], can be represented by a gradient-type transport formula,
[math]Q_{disp} = - A_0 K_x \Large \frac{ds_0}{dx} \normalsize , \qquad(4) [/math]
where [math]s_0[/math] is the tidally averaged salinity concentration, [math]s_0(x)=\lt s(x,t)\gt [/math], [math]K_x [/math] is the longitudinal dispersion coefficient and [math]A_0=\lt A\gt [/math] (the brackets <> represent the average over a tidal cycle). The dispersion coefficient [math]K_x [/math] has the important property that it does not depend explicitly on the salinity distribution in the estuary [2], but only on the flow characteristics during the tidal cycle (which may be influenced by the salinity distribution, by the way). The magnitude of the random displacements depends on the location [math]x [/math] in the estuary; the longitudinal dispersion coefficient [math]K_x[/math] is thus a function of [math]x [/math]. This is illustrated in Fig. 1 for the Eastern Scheldt and Ems-Dollard estuaries.
Longitudinal dispersion mechanisms
Mixing processes, such as those described by the random walk model, cause seawater to intrude further and further into the estuary. However, seawater is also expelled from the estuary due to the inflow of river water at the estuarine head. Seawater intrusion is stabilised when the net upstream salt transport by mixing processes equals the net downstream salt transport by the fluvial discharge. This can be represented by the following formulas.
The fresh water discharge [math]Q_R[/math] is given by
[math]Q_R=-\lt A\overline{\overline{u}}\gt \equiv - \large \frac{1}{T}\int_0^T \int_{-B/2}^{B/2} \int_0^D \normalsize u(x,y,z,t) dzdy dt \qquad(5) [/math]
and the net total salt flux (by mixing processes and river discharge) is given by
[math]Q_S=\lt A\overline{\overline{us}}\gt \equiv \large \frac{1}{T} \int_0^T \int_{-B/2}^{B/2} \int_0^D \normalsize u(x,y,z,t)s(x,y,z,t) dzdydt , \qquad(6) [/math]
where [math]A(x,t)[/math] is the estuarine cross-section, [math]D(x,y,t)[/math] the instantaneous local water depth and [math]B(x,t)[/math] the estuarine width, [math]u[/math] the longitudinal current velocity and [math]s[/math] the salinity. The brackets stand for averaging over the tidal period [math]T[/math] (assuming a cyclic tide) and the overbars stand for averaging over the depth and the width. The coordinate [math]x[/math] follows the upstream positive longitudinal direction (along the thalweg), the coordinate [math]y[/math] the lateral direction and the coordinate [math]z[/math] the upward positive vertical direction.
We call [math] s_0 \equiv \lt \overline{\overline{s}}\gt [/math] the salinity averaged over the estuarine cross-section [math]A[/math] and the tidal period. We may then decompose
[math]Q_S=Q_{disp} - Q_R s_0, \quad Q_{disp} = \lt A\overline{\overline{u(s-s_0)}}\gt \qquad(7) [/math] .
This decomposition singles out the fresh water discharge (the term [math] Q_R s_0[/math] ) as a mechanism for flushing seawater out of the estuary, while the term [math] Q_{disp}[/math] represents the sum of all processes contributing to seawater intrusion. These processes are:
- Horizontal circulations in the estuary (mainly the net relative displacement of water masses circulating between ebb and flood channels and the net relative displacements due to geometry-induced eddies, followed by lateral mixing of these water masses);
- Horizontal tidal straining (lateral mixing between water masses which are advected at different speeds, due to lateral gradients in the longitudinal velocity);
- Vertical circulation in the estuary, also called estuarine circulation (seawater intrusion induced by the density-driven net displacement of near-surface water relative to near-bottom water, followed by mixing over the vertical);
- Vertical tidal straining (vertical mixing between water masses advected at different speeds due to vertical gradients in the longitudinal velocity);
- Lateral mixing of water masses captured in "dead zones" with the main flow;
- Chaotic dispersion, related to the chaotic character of particle trajectories when travelling through a complex field of tide-generated eddies;
- Tidal pumping at the inlet, which is defined here as the partial replacement of the ebb tidal prism with ‘new’ seawater flowing in from the nearshore zone during flood.
Analytical expressions for the longitudinal dispersion coefficient
Under certain simplifying conditions it is possible to derive analytical expressions for the longitudinal dispersion coefficient. These assumptions are:
- the estuarine geometry does not vary strongly in [math]x[/math]-direction over distances comparable to or larger than the tidal excursion;
- the cross-section of the estuarine main channel can be represented approximately a rectangular shape.
We also have the condition [math]T_A\lt \lt T_x[/math], cross-sectional mixing takes place on a time scale much shorter than the estuarine flushing time. In the following we consider different seawater intrusion processes under these conditions and present an approximate analytical expression for the dispersive transport produced by each process.
Dispersion by residual circulation
Fist we consider seawater intrusion caused by estuarine circulation: the up-estuary near-bottom flow caused by the higher density of seawater relative to estuarine water. The estuarine circulation is represented by the velocity component
[math]u_0 (z)= \lt u\gt -\lt \overline{u}^z\gt , \qquad(8) [/math]
where the brackets [math]\lt u\gt [/math] stand for averaging over the tidal period and [math]\overline{u}^z [/math] for averaging over the vertical. The averaging is done in a frame moving with the cross-sectional mean velocity. The longitudinal dispersive transport can be estimated by a procedure outlined by G.I.Taylor [3]. The result is
[math]K_x = f_0^{(z)} T_z \overline{(u_0)^2}^z , \qquad(9) [/math]
with [math] f_0^{(z)} [/math] a coefficient of the order of 0.1 [4]. The formula (9) shows that the dispersion coefficient increases with increasing mixing time [math]T_z[/math]. However, there is a limit, because of the assumption [math]T_z \lt \lt T_x[/math].
For the dispersion coefficient related to lateral horizontal residual circulation a similar formula can be derived, replacing in the expression for [math]K_x [/math] everywhere [math]z[/math] by [math]y[/math]. Expressions for the vertical mixing time [math]T_z[/math] and the lateral mixing time [math]T_y[/math] are given in the section #Time scales for vertical and lateral mixing.
Estuarine circulation is an important seawater intrusion mechanism in estuaries with a single deep (dredged) channel and small to moderate tide. Lateral circulations are important in wide natural estuaries with a complex geometry (meandering main channel , secondary channels, channel bars and tidal flats) and strong tides. The dominance of lateral circulations for seawater intrusion relative to vertical circulations appears in the analytical expression of [math]K_x [/math] through the much larger lateral mixing time [math]T_y [/math] compared to the vertical mixing time [math]T_z [/math]. The presence of distinct ebb and flood channels is a major cause of lateral circulation in wide estuaries, see for example Fig. 2. However, density gradients related to seawater intrusion also produce lateral circulations (see Estuarine circulation), which contribute often even more to longitudinal dispersion than the vertical density-induced circulation [5].
Dispersion by tidal straining
If residual circulations are weak, dispersion is mainly caused by tidal straining, the relative displacement of water masses due to vertical and horizontal gradients in the tidal current velocity. In river flow, the usual term for this mixing mechanism is shear dispersion. Seawater intrusion in narrow deep estuaries is primarily caused by vertical tidal velocity gradients, whereas lateral tidal velocity gradients are important in wide estuaries. We present formulas for vertical tidal straining; the expressions for lateral tidal straining are similar. The process of longitudinal dispersion through tidal straining is explained in Fig. 3.
The velocity component [math]u_1 (z,t) [/math] responsible for vertical tidal straining is
[math]u_1= u-\overline{u}^z , \qquad(10) [/math]
where [math]\overline{u}^z[/math] is the depth-average current velocity. By a procedure outlined by Holley, Harleman and Fischer [6], the following first-order estimate of the longitudinal dispersion coefficient is obtained:
[math]K_x \approx f_1^{(z)} \Large \frac{T_z \lt \overline{ u_1^2}^z\gt }{1+( f_2^{(z)} T_z / T)^2 } \normalsize . \qquad(11) [/math]
The coefficients [math] f_1^{(z)}, f_2^{(z)} [/math] depend on the velocity profile; order-of-magnitude estimates are [math] f_1^{(z)} \sim 0.1-0.2[/math] and [math] f_2^{(z)} \sim 0.5-1 [/math]. [math]T[/math] is the tidal period.
Longitudinal dispersion produced by lateral tidal straining can be expressed by a formula similar as for vertical tidal straining. Dispersion by tidal straining is largest if the time scale for vertical or lateral mixing is on the order of [math]T/ f_2[/math]. The time scale for vertical mixing is generally smaller than the tidal period and the time scale for lateral mixing is generally larger. Dye experiments illustrating dispersion by lateral tidal straining are shown in Estuarine dispersion: dye experiments in the Eastern Scheldt scale model.
It should be realised that longitudinal dispersion is not simply the sum of transport processes related to circulation and straining. Circulation causes not only a net relative displacements of water masses in the estuary, but it also influences tidal straining.
Time scales for vertical and lateral mixing
A difficulty for practical use of the previous expressions for longitudinal dispersion, results from the uncertainty related to estimating the vertical and (especially) lateral mixing times, [math]T_z=D^2/K_z[/math] and [math]T_y=B^2/K_y[/math]. In case of a logarithmic velocity profile (in the absence of density stratification), the vertical diffusion coefficient is given by [math]K_z=0.4z(1-z/D)u_*[/math], where [math]u_*\approx 0.05 U[/math] is the friction velocity and [math]U[/math] the flow velocity. This yields a longitudinal dispersion coefficient [math]K_x \approx 6u_*D[/math], for steady flow [7]. However, in case of buoyant flows, vertical diffusion can be much slower (smaller [math]K_z[/math]), leading to stronger longitudinal dispersion. Lateral diffusion depends strongly on the geometry of the estuary. The lateral diffusion coefficient is generally expressed as [math]K_y \approx \alpha u_* D[/math]. An empirical estimate for moderately meandering channels is [math]\alpha \approx 0.6 [/math] [8] and a model estimate is [math] \alpha \approx 150 (B/ R)^2 [/math] [9], where [math]B[/math] is the channel width and [math]R[/math] is a characteristic channel bend radius.
Dispersion by “dead zones”
The formula for lateral tidal straining may include the influence of "dead zones", if they are considered part of the estuarine cross-section and if they are distributed regularly along the estuary. Dead zones are areas along the main estuarine channel where water is stored during high water, without being significantly transported in longitudinal direction, for instance, tidal flats or lateral creeks (these dead zones should not be confused with the anoxic zones, called 'dead zones' in ecology). The longitudinal dispersion coefficient is given by an expression of the type [10]
[math]K_x=f_{dz} \, r \, U^2T , \qquad(12) [/math]
where [math]U[/math] is the maximum tidal velocity, [math]T[/math] the tidal period and [math]r[/math] is the high-water (HW) storage cross-section of the dead zones relative to the channel cross-section. The coefficient [math]f_{dz}[/math] depends on the mixing rate within the dead zone; in case of complete mixing during the tidal cycle [math]f_{dz}=1/12 \pi^2[/math], assuming that filling of the dead zones starts at low water (LW) [11].
Even without any mixing, storage areas along the channel contribute to longitudinal dispersion, because of a non-zero phase shift [math]\phi[/math] that generally exists between horizontal and vertical tidal motion (i.e., phase shift between [math]u(t)[/math] and [math]d\eta /dt[/math], respectively, where [math]\eta(t)[/math] is the tidal level). We assume dead zones with bed level at low water (or below), which are distributed evenly along the estuarine main channel. Fluid parcels that are stored temporarily in the dead zone experience a net seaward displacement [math]\Delta X[/math] relative to fluid parcels that remain in the channel, see Fig. 4. Filling and emptying of the dead zones during the tidal period then produces a net transport through a plane at [math]x=0[/math] given by
[math]Q_{disp} = \Large \frac{1}{T} \large \int_0^{T/2} \Large \frac{d A_s}{dt} \normalsize dt \large \int_0^{|\Delta X(t)|} \normalsize [s(x,0)-s(0,0)] dx , \qquad(13) [/math]
where [math]A_s(t) = b_s \eta (t) [/math] is the dead zone volume at time [math]t[/math] per unit estuarine length, [math] s(x,t)-s(0,t) \approx x \, ds_0/dx[/math] and [math]\Delta X(t)= -(2U/\omega) \sin \phi \sin \omega t[/math]. The integral evaluates mass transport due to water parcels passing through the plane [math]x=0[/math] during ebb but not during flood (represented by the first term [math]s(x,t)[/math] between the square brackets), taking into account an equivalent landward water flow in the channel (second term [math]s(0,t)[/math] between the square brackets). By evaluating the integral we find for the coefficient [math]f_{dz}[/math] the expression
[math]f_{dz} = \Large \frac{\sin^2 \phi}{3 \pi^2} \normalsize . \qquad (14) [/math]
A usual order of magnitude for the phase shift [math]T\phi/2 \pi[/math] is 30-60 minutes, yielding [math]f_{dz} \approx 0.005 [/math]. In estuaries with large tidal flats dead zones can significantly enhance longitudinal dispersion.
Dye experiments illustrating longitudinal dispersion by dead zones are shown in Estuarine dispersion: dye experiments in the Eastern Scheldt scale model.
In the same way it is possible to estimate the influence of a small lateral creek on the longitudinal dispersion in the estuary. Assuming that filling and emptying of the creek is in phase with the vertical tidal motion [math]d \eta /dt[/math], that the phase difference [math]\phi[/math] with the horizontal tidal motion [math]u(x,t)[/math] is small and that no mixing takes place, the contribution of the tidal creek to the longitudinal dispersion in the estuary is given by [11]
[math]K_x = \sin^2 \phi \, \Large\frac{VL}{2A_0} (1 – (\frac{2x}{L} )^2)\normalsize , \qquad (15) [/math]
where [math]V[/math] is the tidal storage volume of the creek, [math]A_0[/math] is the mean channel cross-section, [math]L=UT/\pi[/math] is the tidal excursion and [math]x[/math] is the along-channel distance to the creek entrance. Equation (15) illustrates why the longitudinal dispersion coefficient can be variable along the estuary. Integration of (15) along the estuary yields the expression (14).
Dispersion by deterministic chaos
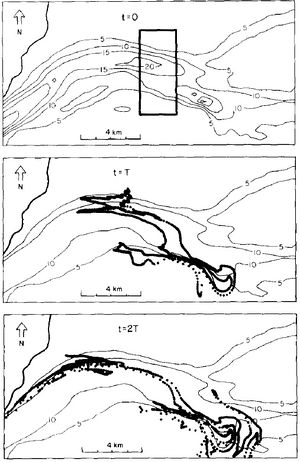
Dye experiments show that dispersion in wide estuaries with complex geometry generally proceeds in an irregular way, by advection of fluid parcels through a field of geometry-induced tidal eddies. The result is very different from turbulent diffusion (i.e. diffusion by a cascade of turbulent eddies of progressively decreasing size). Parts of the dye can be trapped within gyres without any significant turbulent diffusion, while other dye patches can be highly stretched in the flow direction. Strong stretching occurs in particular in the interface zones between tidal eddies, see Fig. 5. Zimmerman (1986) [13] described the dispersion process in such systems as the result of Lagrangian chaos produced by 'a tidal whirlpool'. Fluid parcels can be dispersed over the entire length of the estuary before lateral mixing has taken place. In this case, the random walk description of tidal dispersion is no longer valid. Zimmerman showed that longitudinal dispersion can still be described as a random process, even if turbulent mixing is completely absent. He called this random process “deterministic chaos” [14]. In his model, fluid parcels are dispersed by moving along chaotic orbits through a lattice of tidal eddies. Most dispersion is generated by eddies with a size [math]\lambda[/math] comparable to the tidal excursion length [math]L[/math] [15]. This suggests that the longitudinal dispersion coefficient for chaotic mixing should be proportional to
[math]K_x \propto UL . \qquad(16) [/math].
The size of the eddies also depends on the basin with [math]B[/math]. A field study in Willapa Bay (US Pacific coast) suggests that chaotic dispersion could be described by a dispersion coefficient [math]K_x = 0.06 UB[/math] [16]. If the lateral mixing time [math]T_y[/math] is comparable to or larger than the flushing time [math]T_x [/math], the representation of the dispersive transport [math] Q_{disp}[/math] by the product of a dispersion coefficient and the local salinity gradient is no longer valid.
Dispersion by tidal pumping
Salt intrusion by tidal pumping is related to the higher average salinity of seawater entering the estuary during flood compared to the average salinity of estuarine water flowing out into the sea during ebb. It depends on the rate of renewal of estuarine water in the outflow region and therefore on tidal inflow-outflow hydrodynamics. Outflow of estuarine water often has a jet-like character, whereas flood water enters the estuary more distributed from different directions, depending on the tidal phase, see Fig. 6. The inflowing flood water therefore contains ‘new’ seawater from the sides of the ebb channel. Replacement of outflowing estuarine water by "new" seawater is enhanced when river discharge is high, because the ebb jet is concentrated in a surface layer (salinity stratification), whereas seawater inflow is more evenly distributed over the vertical.
We call [math]\alpha[/math] the replacement rate of outflowing estuarine water by seawater during a tidal cycle. For small fresh water discharge, the corresponding dispersion coefficient at the estuarine mouth is given by
[math]K_{mouth} = \alpha \Large \frac{L^2 }{2T} , \normalsize \qquad(17) [/math]
where [math]L=\int_{flood} u(t)dt [/math] is the tidal excursion at the estuarine mouth. Savenije [17] derived the following empirical expression for [math]\alpha[/math], based on observations suggesting that the renewal of estuarine water in the mouth area depends on the buoyancy of the ebb jet:
[math]\alpha=\Large \frac{2800 \pi D}{l_A} \normalsize \sqrt{Ri_E} . \qquad(18) [/math]
In this expression [math]D[/math] is the depth and [math]l_A[/math] the convergence length of the estuary in the outflow zone (assuming exponential convergence of the cross-section in the outflow zone, [math] A(x) \sim exp(-x/l_A)[/math]);
[math]Ri_E[/math] is the Richardson estuary number [math]Ri_E=g \Delta \rho Q_R T^3 / ( \pi^2 \rho B L^3)[/math];
[math] \Delta \rho / \rho[/math] is the relative density difference seawater-fresh water and [math]B[/math] the estuarine width at the mouth. The value of [math]\alpha[/math] should not exceed 1. Tidal pumping is a major mechanism of seawater intrusion in estuaries during periods of high river flow.
Residence time scale
The residence time [math]T_r[/math] is defined as the average time a water parcel, which is initially located at a distance [math]x[/math] from the sea boundary, will take to leave the estuary. If the fresh water discharge is zero or very small, and if the dispersion coefficient [math]K_x[/math] is assumed constant along the estuary, the residence time is given by random walk theory [18]:
[math]T_r = \Large \frac{x^2}{2K_x} \normalsize . \qquad(19) [/math]
The flushing time [math]T_f=T_x[/math] is the average time it takes for a fresh water parcel to move through the estuarine zone to the sea. According to the random walk model, for small discharge [math]Q_R[/math],
[math]T_f = \Large \frac{l^2}{2K_x} , \normalsize \qquad(20) [/math]
where [math]l[/math] is the estuarine length. This is approximately equivalent to [math]T_f = V_f / Q_R[/math] , where [math]V_f[/math] is the fresh water volume in the estuary.
Experimental determination of the longitudinal dispersion coefficient
The dispersion coefficient [math]K_x[/math] can be determined experimentally in situations where the freshwater discharge [math]Q_R[/math] is constant over a period longer than [math]T_x[/math]. In that case the salinity distribution [math]\lt s(x,t)\gt [/math] is close to equilibrium ([math] \lt s(x,t)\gt \approx s_0(x) [/math]). The total residual salt flux [math]Q_S[/math] approximately equals zero. We thus have (with the sign convention [math]Q_R \gt 0[/math])
[math]AK_x \Large \frac{ds_0}{dx} \normalsize + Q_R s_0 = 0 . \qquad(21) [/math]
Values of the dispersion coefficient can be derived from measurements of the residual discharge [math]Q_R[/math] and the salinity distribution [math]s_0(x)[/math]. Estimates of longitudinal dispersion coefficients determined in this way are shown in Table 1. More detailed analyses show that the dispersion coefficient [math]K_x[/math] is a function of [math]x[/math] and [math]Q_R[/math]. The dependence of [math]K_x[/math] on [math]Q_R[/math] has two causes. It is related in the first place to the influence of the salinity distribution on the velocity flow field [math]u(x,u,z,t)[/math]; such an influence is due to salinity-induced density gradients (see Estuarine circulation). In the second place, it is related to the location of the freshwater-seawater transition zone. If this zone is situated near the estuarine mouth, the dispersion coefficient is strongly influenced by tidal pumping. This explains the high longitudinal dispersion coefficients for Rotterdam Waterway, Seine and Loire in Table 1, which are determined for situations with important river flow [math]Q_R/hb[/math]. The same holds, to a somewhat lesser degree, for the Elbe, Weser, Mekong and Sinnamary.
The influence of salinity-induced density gradients on the longitudinal dispersion coefficient is generally small in estuaries with complex topography and small river inflow ([math]Q_R/hb[/math] several orders of magnitude smaller than the tidal velocity). This is the case for many tidal lagoons, such as the Wadden Sea.
If a non-buoyant dissolved substance is introduced in the estuary, it will be mixed over the cross-section after a time [math]T_A[/math] . From that time, the longitudinal dispersion of the substance is similar to salinity dispersion (same dispersion coefficient). For a permanent discharge of a non-buoyant dissolved substance, the same dispersion coefficient applies in the estuarine zones where the substance is mixed over the estuarine cross-section.
| estuary | tidal range [math]2a[/math] [m] | depth [math]D[/math] [m] | width [math]B[/math] [km] | discharge [math]Q_R[/math] [m3/s] | dispersion coefficient [math]K_x[/math] [m2/s] |
|---|---|---|---|---|---|
| Bay of Fundy (Canada) | 10 | 20 | 20 | 150 | 300 |
| Bristol Channel (UK) | 8 | 30 | 20 | 480 | 60 |
| Chao Phraya (Thailand) | 2.5 | 7.2 | 0.6 | 30 | 330 |
| Corantijn (Surinam) | 4.3 | 6.5 | 3 | 500 | 230 |
| Delaware (US) | 1.5 | 6.6 | 7 | 300 | 300 |
| Eastern Scheldt (Netherlands) | 3 | 12.5 | 2 | 60 | 200 |
| Elbe (Germany) | 3.3 | 12 | 2.5 | 750 | 700 |
| Ems-Dollard (Netherlands) | 3 | 9 | 4 | 100 | 275 |
| Gambia (The Gambia) | 1.2 | 8.7 | 4 | 2 | 200 |
| Hudson (US) | 1.6 | 11,6 | 2.2 | 100 | 110 |
| Humber (UK) | 5.5 | 12 | 3 | 250 | 300 |
| Incomati (Mozambique) | 5.5 | 2.9 | 0.6 | 1 | 10 |
| Limpopo (Mozambique) | 2.6 | 7 | 0.2 | 5 | 150 |
| Loire (France) | 4.5 | 9 | 0.9 | 825 | 900 |
| Mae Klong (Thailand) | 2 | 5.2 | 0.2 | 30 | 200 |
| Maputo (Mozambique) | 6.7 | 3.6 | 1.3 | 10 | 100 |
| Mekong-Co Chien+Cung Hau (Vietnam) | 2.1 | 7 | 4 | 2125 | 570 |
| Mekong-Tran De+Dinh Anh (Vietnam) | 2.8 | 8 | 3 | 2250 | 530 |
| Mersey (UK) | 7.5 | 20 | 1 | 80 | 400 |
| Potomac (US) | 1.4 | 8.4 | 9 | 110 | 70 |
| Pungue (Mozambique) | 5 | 11.5 | 1.8 | 20 | 140 |
| Rotterdam Waterway (Netherlands) | 0.9 | 15 | 0.6 | 1000 | 1000 |
| St. Lawrence (Canada) | 3 | 74 | 48 | 8500 | 200 |
| Seine (France) | 5.5 | 8 | 0.8 | 440 | 800 |
| Sinnamary (Guiana) | 2.3 | 3.8 | 0.3 | 100 | 560 |
| Solo (Indonesia) | 1.1 | 9.2 | 0.17 | 10 | 240 |
| Tha Chin (Thailand) | 2.9 | 5.3 | 0.2 | 10 | 270 |
| Thames (UK) | 4.5 | 12 | 3 | 60 | 100 |
| Weser (Germany) | 3.8 | 9 | 2 | 324 | 1000 |
| Western Scheldt (Netherlands) | 3.8 | 16 | 3.5 | 100 | 200 |
Related articles
Estuarine dispersion: dye experiments in the Eastern Scheldt scale model
Physical processes and morphology of synchronous estuaries
References
- ↑ Taylor, G.I., 1921, Diffusion by Continuous Movements. Proc., London Math. Soc., Ser. A 20: 196-211
- ↑ Dronkers, J. 1982. Conditions for gradient-type dispersive transport in one-dimensional tidally averaged transport models. Est.Coast.Shelf Sci. 14: 599-621
- ↑ Taylor, G.I. 1954. The dispersion of matter in turbulent flow through a pipe. Procs. Royal Society Londin A223: 446-468
- ↑ Fischer, H.B., List, E.J., Koh, R.C.Y., Imberger, J. and Brooks, N.H. 1979. Mixing in Inland and Coastal Waters. Academic Press, New York
- ↑ Smith, R. 1980. Buoyance effects upon longitudinal dispersion in wide well-mixed estuaries. Philos. Trans. Royal Soc. London A 296: 467-496
- ↑ Holley, E.R., Harleman, D.R. and Fischer, H.B. 1970. Dispersion in homogeneous estuary flow. J. Hydr. Div. ASCE 96: 1691-1706
- ↑ Elder, J.W. 1959. The Dispersion of Marked Fluid in Turbulent Shear Flow. J. Fluid Mech. 5: 544-560
- ↑ Fischer H.B. 1972. Mass transport mechanisms in partially stratified estuaries. J. Fluid Mech. 53: 671–687
- ↑ Yotsukura, N. and Sayre, W.W. 1976. Transverse mixing in natural channels. Water Resources Res. 12: 695–704
- ↑ Okubo, A. 1967. The effect of shoreline irregularities on horizontal diffusion from an instantaneous source. Inter. J. Oceanol. Limnol. 1: 194-204
- ↑ 11.0 11.1 Dronkers, J. 1978. Longitudinal dispersion in shallow well-mixed estuaries. Procs. 16th Int. Conf. Coastal Eng. 3: 2761-2777
- ↑ Ridderinkhof, H., Zimmerman, J. T. F. 1990. Mixing Processes in a Numerical Model of the Western Dutch Wadden Sea. In: Coastal and Estuarine Studies, Vol. 38 Residual Currents and Long·term Transport, R. T. Cheng (Ed.), Springer
- ↑ Zimmerman, J.T.F. 1986. The Tidal Whirlpool: A Review of Horizontal Dispersion by Tidal and Residual Currents. Neth. Journal of Sea Research 20:133-154
- ↑ Ridderinkhof, H. and Zimmerman, J. T. F. 1992. Chaotic stirring in a tidal system. Science 258: 1107-1109
- ↑ Zimmerman, J.T.F. 1976. Mixing and flushing of tidal embayments in the western Wadden Sea, I: Distribution of salinity and calculation of mixing time scales. Neth.J.Sea Res. 10:149-191
- ↑ Banas, N.S., Hickey, B.M., 2005. Mapping exchange and residence time in a model of Willapa Bay, Washington, a branching, macrotidal estuary. Journal of Geophysical Research 110, C11011. doi:10.1029/2005JC002950
- ↑ Savenije, H. H. G. 2005. Salinity and Tides in Alluvial Estuaries. Elsevier, Amsterdam, 197 pp.
- ↑ Zimmerman, J.T.F. 1981 The flushing of well mixed tidal lagoons and its seasonal fluctuations. UNESCO Tech. Pap. Mar. Sci. 33: 15-26
- ↑ Prandle, D. 2004. Saline intrusion in partially mixed estuaries. Estuarine, Coastal and Shelf Science 59; 385-397
- ↑ Vandenbruwaene, W., Plancke, Y., Verwaest,T. and Mostaert F. 2013. Interestuarine comparison: Hydro-geomorphology Hydro- and geomorphodynamics of the TIDE estuaries Scheldt, Elbe, Weser and Humber. TIDE Report Flanders Hydraulics Research WL 2013-770
- ↑ Van Rijn, L. 2011. Comparison Hydrodynamics and Salinity of Tide Estuaries: Elbe, Humber, Scheldt and Weser. TIDE Report, Deltares 1203583-000
- ↑ Holloway, P.E. 1981. Longitudinal Mixing in the Upper of the Bay of Fundy Reaches. Estuarine, Coastal and Shelf Science I3: 495-515
- ↑ Helder, W. and Ruardij, R. 1982. A one-dimensional mixing and flushing model of the Ems-Dollard estuary: calculation of time scales at different river discharges. Neth. J. Sea Res. 15: 293-312
- ↑ Nguyen, A.D. 2008. Salt intrusion, tides and mixing in multi-channel estuaries. PhD thesis Delft University
- ↑ Publications GIP Seine-aval http://www.seine-aval.crihan.fr/ and GIP Loire-estuaire http://www.loire-estuaire.org/
Please note that others may also have edited the contents of this article.
|