Input-output matrix
An Input-output matrix is a representation of national or regional economic accounting that records the ways industries trade with one another as well as produce for consumption and investments.
Contents
Introduction
An input-output matrix is a table that shows how different sectors of the economy are connected. It helps explain where goods and services come from, where they go, and how one industry depends on another (OECD, 2006[1]; Eurostat, 2008[2]).
The basic idea is simple: the output of one sector often becomes the input of another sector. For example, agriculture produces cotton. The textile industry buys this cotton and uses it to make clothes. In this case, cotton is the input, and clothes are the output.
This relationship between industries is called intermediate demand. It refers to goods and services that firms buy from other firms and use in their own production process. For example, when a textile factory buys cotton from farmers, this is an intermediate transaction. These exchanges are shown in the Domestic Intermediate Matrix (the first quadrant of Table 1).
Once goods and services are produced, they are also sold to users outside the production process. This is called final demand. Final demand includes:
- households buying goods and services for daily consumption,
- businesses investing in machines, buildings, and equipment,
- government spending on public services,
- exports sold to other countries.
These purchases are called final demand because they are not used to produce other goods (Common and Stagl, 2005[3]). They represent the final use of production. This is shown in the Final Demand Matrix (the fourth quadrant of Table 1).
In addition to buying goods from other firms, companies also need primary inputs, also called factors of production. These are resources provided by people rather than by firms. They include:
- labour (paid through wages and salaries),
- land and buildings (paid through rent),
- capital such as borrowed money (paid through interest),
- entrepreneurship and business risk-taking (rewarded through profit).
These primary inputs create what economists call value added, which is an important part of production. They are shown in the second quadrant of the input-output Table 1.
The full input-output matrix contains several other quadrants that show these different flows between producers and final users. Some sections are more important for understanding the basic method than others, but the main purpose remains the same: to show how sectors of the economy depend on each other.
This method is useful for studying economic structure, trade between industries, employment effects, environmental impacts, and policy decisions. It helps economists and decision-makers understand how changes in one sector can affect many others across the whole economy.
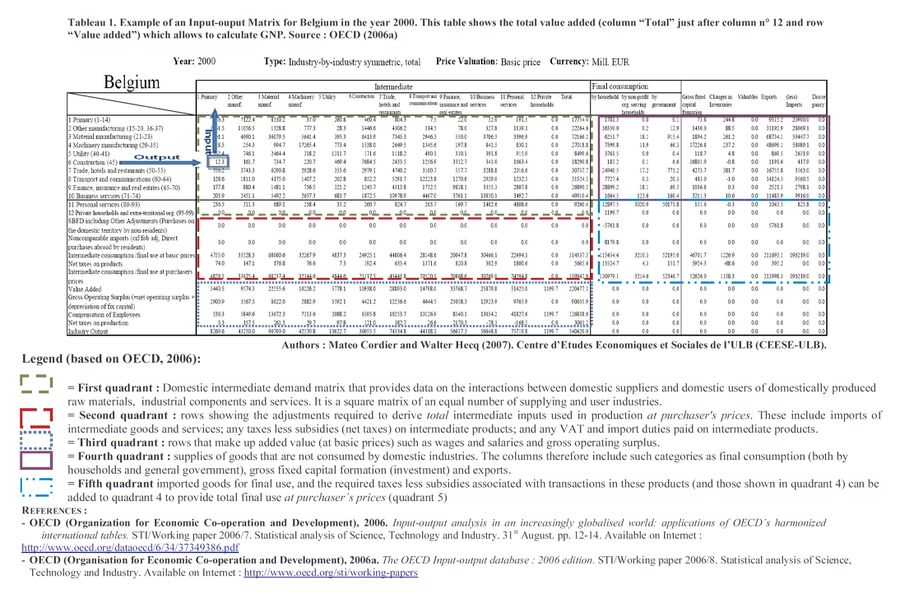
Table 1 shows an example of a real input-output matrix for Belgium in the year 2000. The columns represent the destination of inputs, and the rows sum the output of a sector.
As you can see, only the total outputs (last line called industry output) are shown, not the total inputs. This is because such data is not necessary since the total inputs equal the total outputs. Normally, this total appears in the last column of the table.
Practical use of Input-output tables
Input-output matrices are useful because they help us understand how different parts of the economy are connected. They show how a change in one sector can affect many other sectors.
For example, if demand for fish increases, the fishing industry may need more fuel, equipment, transport, and labour. This creates effects in other sectors such as energy, manufacturing, and services. In this way, the input-output matrix helps trace both direct and indirect economic effects.
This method is often used to study:
- the economic importance of a sector,
- employment effects of new investments,
- environmental impacts such as energy use or pollution,
- the effects of tourism, fisheries, transport, or coastal development,
- national and regional economic planning.
This can be particularly appreciable for assessing economic impact (both ex-ante and ex-post) of policy changes. Environmental impact can even be analyzed if we add environmental data to classical input-output tables in order to build green input-output tables.
It is also useful for policy-making because it helps governments and researchers estimate how economic changes in one activity may influence the wider economy. For instance, if a policy option scenario for marine pollution management (e.g. a tax on plastic industry resulting in higher plastic prices or a governmental subsidies to the production of material of substitution that are biodegradable) results in technical changes or in changes in final demand for plastics (a valuable material particularly in construction, packaging and fishing gear applications), I-O analysis can help us to deduce the following (adapted from Leontief, 1974[4]):
- the policy options impact on the total level of pollution by plastic microparticles in the sea
- the amount of pollution reduction in a particular sector resulting from the implementation of a policy option
- the total pollution resulting from the final demand (demand from households, …) for products of each sector. For instance, keeping the example of plastic production, this means that the I-O table can tell us : “from the total amount Y of plastic pollutant in the sea, X tones are linked to agriculture, industrial and services activities contributing directly or indirectly to the supply of agricultural products to households. This is interesting since it does not only take into account the amount of pollutants from the agriculture sector for the production of agricultural products, but it also encompasses pollutants from other sectors intervening in the production of agricultural products. That is important since the agriculture sector also needs industrial products and services to generate its production. The same can be calculated for the supply of industrial products and services to households.
- the impact of policy options on production level in other sectors (and so on the economy)
- the impact of policy options on total employment in the region or in a particular sector
- the impact of policy options on prices of goods and services
Limits of the method
Although input-output matrices are very useful, they also have some important limitations.
The input-output (I-O) analysis is not able to capture environmental measures with a small economic impact (on GDP, on production, on employment…at national or regional level) because of data are too aggregated. Therefore, I-O is only relevant for activities having a wide economic impact such as construction of large infrastructures (railways or motorways infrastructure), modification of port activities, implementation of environmental policies targeting a whole sector, subsector or a branch of economic activities, etc.
Nevertheless, I-O analysis could also be relevant for a package of several policy options, each having a relatively small impact, but whose sum results in a large impact on the regional economy.
Walter Hecq (2006a[5]) summarized several other limits of I-O analysis. They are mentioned below.
First, they assume that production methods do not change. This means the model assumes that firms always use the same amount of inputs to produce the same amount of output. In reality, companies can change technology, improve efficiency, or use different materials.
Second, prices are usually assumed to stay constant. The model does not easily capture changes in prices, inflation, or market competition.
For example, it is not possible to change between production factors (labour, technical capital, land) while environmental policies might precisely have a structural effect on the long run on that aspect. Take the example of an environmental policy aiming at decreasing greenhouse gases emissions by promoting research and development in energy efficiency in households. Suppose the instrument of this policy would consist in public subsidies to universities for research in building insulation new technologies. Such a measure might lead to reduction of households energy consumption and so a reduction in natural gas extraction burnt in power plants for electricity generation. In that case, the production factor “land” in the form of a natural resource (natural gas) has been partly substituted by the production factor “labour” (development of human knowledge in new insulation techniques).
Third, input-output tables are often based on large amounts of national data, which can be expensive and time-consuming to collect. Because of this, the information may become outdated quickly.
Finally, the model gives a simplified picture of the economy. It shows relationships between sectors clearly, but it cannot fully explain behaviour such as consumer choices, innovation, or unexpected economic shocks.
For these reasons, input-output analysis is best used as a tool for understanding economic structure and estimating general effects, rather than for making exact predictions.
Related articles
- Supply chain analysis
- Computable general equilibrium
- Green accounting
- Regional economic accounting methods
- Multifunctionality and Valuation in coastal zones: concepts, approaches, tools and case studies
- Multifunctionality and Valuation in coastal zones: introduction
References
- ↑ 1.0 1.1 Wixted, B., Yamano, N. and Webb, C. 2006. Input-Output Analysis in an Increasingly Globalised World:Applications of OECD's Harmonised International Tables, OECD Science, Technology and Industry Working Papers, 2006/07, OECD Publishing.
- ↑ Eurostat Manual of Supply, Use and Input-Output Tables
- ↑ Common M., Stagl S. 2005. Ecological Economic. An Introduction. Cambridge University Press, New York, pp.125-136
- ↑ Leontief V., 1974. Essais d’économiques. Ed. Calman Lévy, pp.133-157 and 193-216. Those two chapters are also available in English in :
- Input-output Analysis, Input-output Economics, New York Oxford University Press, 1966;
- Environmental repercussions and the Economic Structure : An Input-Output Approach, published in The Review of Economics and Statistics, Vol. LII, n°3, August 1970, Copyright by the president and Fellows of Harvard College; published as well in Robert et Nancy DORFMAN, Economics of the Environment, W.W. Norton & Co Inc, 1972.
- ↑ Hecq W., 2006a. Aspects économiques de l’environnement. Fascicule 4. Economie de l’environnement. Université Libre de Bruxelles, 12ème édition, P.U.B.
Please note that others may also have edited the contents of this article.
|
