Shelf sea exchange with the ocean
Introduction
Deep ocean basins have typical depths of 4000 m or more. However, continents are typically surrounded by continental “shelf” above which the depth of the sea is typically 0-200 m. At the edge of the shelf, there is a steep slope down to oceanic depths in most places. The extent of the shelf (sea) varies from zero around atolls and volcanic sea mounts (for example) to tens of kilometres typically but hundreds of kilometres off NW Europe, Argentina and around much of the Arctic Ocean (Figure 1), see also Open ocean habitat.
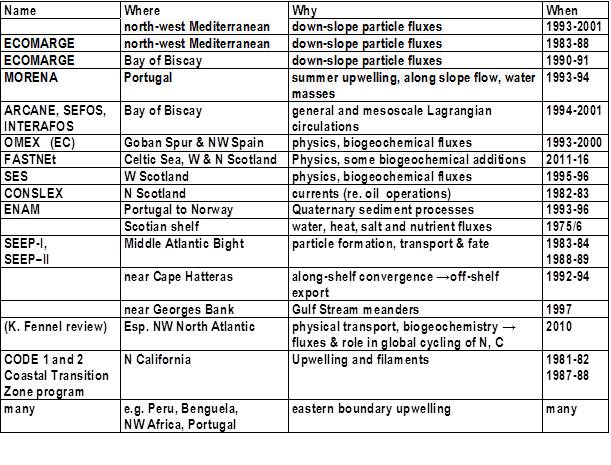
Questions of global cycling entail the quantity, transformation and fate of materials carried between the shelf seas and ocean, and hence processes of ocean-shelf transport and exchange. For example, transport from the open ocean across the shelf edge is estimated to bring most of the nitrogen and half of the phosphate used in global shelf-sea export production [1]; these transports support the shelf seas’ enhanced primary production and thence 90% of the world’s commercial fish catch [2]. Hence many ocean-shelf sea interaction studies have taken place (Table 1 lists some), illustrating strong biogeochemical interests but necessary physics underpinning.
Physical processes control the large-scale movement and irreversible small-scale mixing of water and its constituents. At the shelf edge, steep bathymetry may inhibit ocean-shelf exchange: integration of the momentum equation through depth and around a depth contour shows that net cross-contour transport is entirely attributable to ageostrophy (Huthnance[3], see also Geostrophic flow). That is, transport across depth contours is enabled by (i) time scales of a day or less, or (ii) space scales of a few kilometres or less, or (iii) depth contours changing direction on similarly short space scales or (iv) enough friction to stop the flow in a day or so.
The combination of sloping topography and stratification gives rise to shelf-edge processes satisfying one or more of the criteria (i-iv). These include coastal-trapped waves; instability, meanders and friction-induced Ekman transport associated with along-slope currents; eddies; upwelling, fronts and filaments: down-welling, cascading; tides, surges; internal tides and waves; surface waves; topography (capes and canyons).
In view of the complex set of processes, often complex topography and the small scales favouring cross-slope flow, our approach to better representation of ocean-shelf interaction is to develop models with fine resolution (of order 1 km). These then need testing against detailed measurements in areas with contrasting conditions (hence varied processes).
The above processes occur with varying intensity according to context, but some (e.g. wind-induced upwelling or down-welling, tides) enable cross-slope transports of order [math]1 \; m^2/s[/math] or more (per metre of shelf edge). This is small compared with transports of order [math]100 \; m^2/s[/math] (per metre width) in strong ocean currents such as the Gulf Stream. However, the global length of shelf edge, [math]O(5 \times 10^5 km)[/math] [4], gives a global aggregate cross-slope transport probably exceeding the transport in any ocean current.
In the following, typical estimates of exchanges are given in [math]m^2/s[/math]: volume per second across a 1 m sector of shelf edge. These are the same units as for dispersion coefficient [math]K[/math], a different quantity but related: [math]K dC/dx[/math] or [math]K \Delta C / L_x[/math] represents transport [math]|u'C'|[/math] due to unresolved fluctuations of current [math]u'[/math] and constituent [math]C'[/math]. Thus:
[math]K \sim | u'| L_x[/math] and exchange [math] |u'|h/2 \sim Kh/2L_x \qquad (1) , [/math]
with [math]h, \; L_x[/math] respectively the depth at the shelf edge and the cross-shelf scale (e.g. the shelf width). If as occasionally below we estimate [math]K[/math], then a (dimensionless) factor [math]h/2L_x[/math] is applicable to estimate the associated exchange.
Processes
Coastal-trapped waves
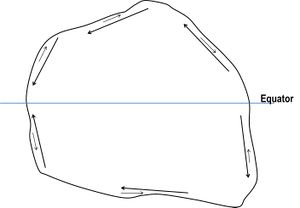
These are the basic waves that travel along the continental shelf and slope. Scales are typically one to several days and tens to hundreds of kilometres according to the width of the continental shelf and slope. The lowest mode (0) ‘Kelvin’ waves, also coastally trapped, travel cyclonically around ocean basins but with typical scales of thousands of kilometres both alongshore and for offshore decrease of properties.
Coastal-trapped waves have been widely observed, along coastlines of various orientations and all continents in both the Northern and Southern Hemispheres. Mode 1 with simple structure (usually one offshore zero of bottom pressure; figure 2 left panel) has been most often identified; its peak coastal elevation is relatively easily measured. Higher modes (with more offshore zeros; figure 2 right panel) have been identified off Oregon, the Middle Atlantic Bight and New South Wales (Australia).
Coastal-trapped waves are not an independent cause of transports; their significance is in propagating shelf-wide day-to-day variations. Examples are:
- oceanic tides with large coastal sea-level variations and (on broad shelves) large currents;
- storm surges; strong currents and large changes of sea level induced by weather (atmospheric pressure and especially winds);
- wind-forced upwelling;
- along-slope currents and poleward undercurrents common on the eastern sides of oceans;
- responses to oceanic eddies and alongshore pressure gradients.
Thus transports induced in one location may appear later at another. The sense of phase propagation is cyclonic around an ocean basin (anti-clockwise in the northern hemisphere; clockwise in the southern hemisphere; figure 3). However, short-wave energy may travel slowly in the opposite sense, especially if stratification is weak [3].
Along-slope currents

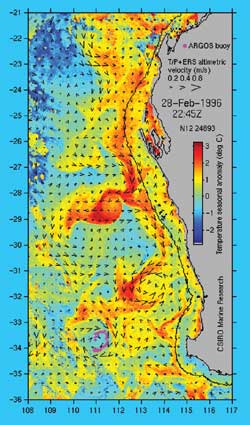
Western boundary currents such as the Agulhas, Brazil Current, Gulf Stream and Kuroshio can be strong, sufficient to form meanders and eddies in some locations (e.g. Gulf Stream Rings; figure 4). They may also extend to the bottom to give a frictional cross-slope Ekman transport. Reviews of the contribution of Gulf Stream Warm-Core Rings to shelf/slope water exchange [5][3] suggest an average [math]O(0.5 \; m^2/s)[/math] north-east of the separation of the Gulf Stream from the shelf.
Along-slope currents are common at eastern ocean margins [6]. Equatorward surface currents are associated with upwelling in the north and south Pacific and Atlantic. Poleward flows over the upper continental slope occur off western USA, western Australia (figure 5) and western Europe, for example, forced by alongshore gradients in adjacent ocean density. In upwelling areas such poleward flow may be an undercurrent not showing at the surface.
Upwelling is a consequence of wind-forced off-shelf surface Ekman transport [math]\tau_w / \rho f .[/math] (Here [math]\tau_w[/math] is wind stress, [math]\rho[/math] is sea density and [math]f[/math] is Coriolis parameter.) The associated along-shelf flow may become strong enough to go unstable and develop meanders and filaments (figure 6); these enhance cross-shelf transport beyond [math]\tau_w / \rho f .[/math] In any case, flow at the bottom results in a bottom stress [math]\tau_b[/math] and corresponding Ekman transport [math]\tau_b / \rho f .[/math] which is off-shelf under poleward flow and on-shelf under equatorward flow.
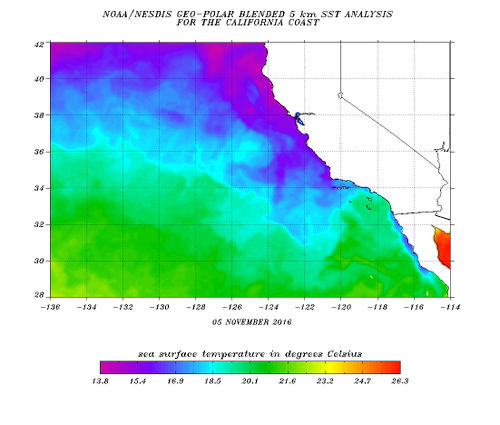 Figure 6: Upwelling of California with meanders and filaments shown by cooler sea-surface temperature. At http://oceansjsu.com/105d/exped_climate/10.html |
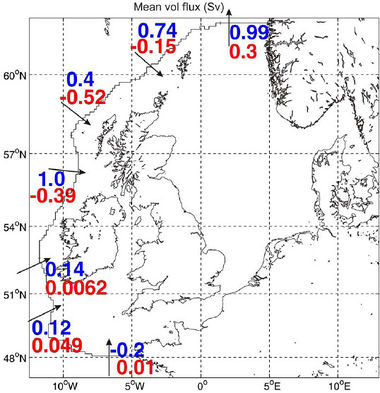 Figure 7: Fluxes (Sv) above 150m (blue) and below 150m depth (red). All fluxes are across the 200m contour shown; positive is onto the shelf except next to Norway (positive to north). From Huthnance et al.[7] |
Given a typical drag coefficient 0.00125 for wind stress over the sea [8] and 0.0025 for currents above the sea bed [9], these Ekman transports are about [math]1 \; m^2/s[/math] for a typical wind of 8 m/s or near-bed current 0.2 m/s. For example, modelled 1960-2004 mean down-welling circulation for the north-west European shelf from Brittany to the Norwegian Trench was about 1.2 Sv [7] (figure 7) as a result of winds driving surface waters onto the shelf and bottom frictional Ekman transport off the shelf [math](1 Sv= 10^6 \; m^3/s).[/math] The latter is associated with prevailing poleward flow of order 2 Sv along the continental slope (typically in 200 to 1000 m depth) around Ireland and Scotland.
Cascading
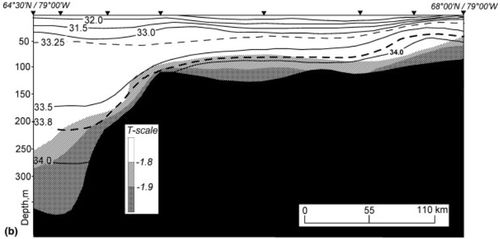
Dense water formed by winter cooling of shallow shelf seas may cascade down the slope under gravity (figure 8), eventually leaving the sloping bottom at its density level. [The excess density may be enhanced if sea ice forms, adding salt to the unfrozen water. Increased density resulting from salination through evaporation can also occur in hot dry conditions.] Typical values of cascading fluxes are estimated as [math]0.5-1.6 \; m^2/s[/math] [12], significant when and where they occur but highly intermittent.
Tides
Tides are a consequence of gravitational forces on the solid earth and especially on the ocean. The forcing is global in scale and mainly acts on wide ocean basins. However, the largest tidal elevations are seen at coasts bounding broad shelf seas, where tidal currents are strongest and much of the global tidal energy dissipation occurs. Thus tides are an example of full ocean – shelf sea interaction, with further amplification on broad shelves, see Ocean and shelf tides and Tidal motion in shelf seas.
Consider a uniform shelf of width [math]W[/math] and semi-diurnal tidal range [math]R[/math]. A volume [math]WR[/math] per unit length has to be supplied in 6.2 hours to fill the “tidal prism” between low and high tide. If sinusoidal in time, the required tidal current across the shelf edge in depth [math]h[/math] reaches [math]\pi WR/hT[/math] ([math]T[/math] is the semidiurnal tidal period). For example, the Celtic Sea (continental shelf) south-west of the UK is up to 400 km wide with large tidal range, e.g. 4 m at Newlyn. [math]\pi WR/hT[/math] with [math]h[/math] = 150 m gives about 0.75 m/s. Thus in places the peak ebb and flood currents exceed 0.5 m/s; the corresponding exchanges are as much as [math]80 \; m^2/s.[/math]
However, semi-diurnal tidal exchanges are reversed every 6.2 hours; this is too soon for much to happen in the water, reducing their effect. Long-term exchange is associated with tides through shear dispersion, coefficient about [math]10^3|U^2|[/math] or [math]500 \; m^2/s[/math] [13]. From (1) we estimate the associated exchange as [math]0.1 \; m^2/s[/math] or more [math](h= 150 \; m, \; L_x \le 400 \; km).[/math]
Surges
This refers to flows driven by weather systems so that spatial scales typically correspond to the width of shelf and time-scales are several hours to a day or two. Shallow shelf seas are relatively easily accelerated. Hence mode 1 coastal-trapped waves tend to be generated on account of their matching scales [3]. Thus longer-period wind forcing tends to be associated with along-slope flow; higher frequencies break the geostrophic constraint to allow cross-slope flow, especially near a mode-1 frequency maximum for which the wave tends to have anti-cyclonically polarised flow near the shelf edge. Otherwise surges tend to be over the shelf, not in deeper water, with maximum elevation at the coast.
Internal tides and waves
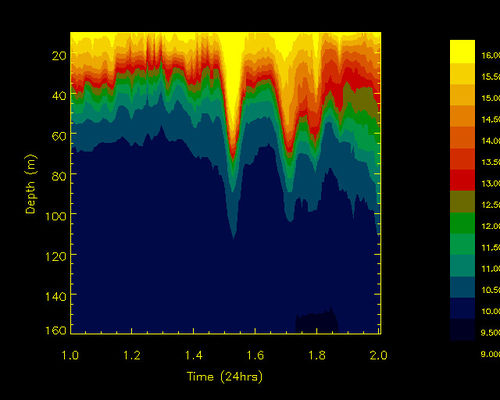
Internal tides are formed when shelf-edge topography causes vertical displacements in cross-slope tidal flow. If they were entirely linear, then no net transport would result during a tidal cycle. However (i) layer thickness may correlate with oscillatory velocity and (ii) strong cross-slope tidal currents generate large-amplitude internal tides.
(i) For a single layer or a surface wave, this correlation implies an ‘eddy’ transport equivalent to the Stokes Drift. For multiple layers it forms a component of the Stokes Drift. Such ‘eddy’ transport is liable to be offset by opposing mean flow [math]\hat u[/math] in the same layer or elsewhere.
(ii) If vertical displacements are a substantial fraction of water depth, then the motion is non-linear, the waves steepen and form one or more solitons (figure 9) which carry water “bodily” as part of the wave form (e.g. Celtic Sea [15], South China Sea [16]). Then the transport can be estimated from the soliton amplitude, length and speed; up to [math]1 \; m^2/s[/math] in extreme cases such as the Celtic Sea, NW Australia and Georges Bank (Huthnance[3], reviewing several authors’ work).
Internal waves occur at all frequencies [math]\sigma[/math] from the Coriolis frequency [math]f[/math] to the buoyancy frequency [math]N[/math], and all wave directions. They occur throughout the ocean from wind and tidal forcing; their oceanic spectrum is empirically near-universal except close to sources [17]; the energy density corresponds to an estimated shelf-ward energy fIux of the order of 1 kW/m [18]. Their strong vertical and horizontal currents, and breaking in places, cause turbulent mixing affecting many ocean processes. If the sea-floor slope closely matches the waves’ characteristic slope [math][(\sigma^2-f^2)/(N^2-\sigma^2)]^{1/2}[/math] then the currents may be amplified several times within a bottom boundary layer.
The shelf edge provides an effective source of internal waves: at tidal frequencies as above with higher-frequency contributions if non-linearities are significant; as standing lee waves in longer-period flow along a rough continental slope [19] and analogously around a seamount [20]. Moreover, internal wave motion along characteristics is reflected off the sloping bottom, with a change of wavelength. Internal wave energy may also transmit to the shelf; but reflection is strong if the bottom slope exceeds the characteristic slope for the wave [21]. The most energetic known internal waves are generated in Luzon Strait (NE South China Sea) and cause turbulence exceeding 10,000 times background ocean turbulence [22]
Surface waves
These may contribute to circulation and exchange via non-linear rectification of their currents. Estimated surface wave currents [23] are [math]0.015 w - 0.035 w[/math] under wind speed [math]w[/math], decaying with depth on a scale [math]w/3[/math] in mks units. The Stokes Drift (Lagrangian – Eulerian) transport, [math] \lt (\vec X .\vec \nabla) \vec u \gt \sim 0.01 \; w^2[/math] [where [math]\vec X = \int \vec u dt[/math]] is thus comparable with the Ekman transport [math]\tau/ \rho f \sim 0.01 \; w^2[/math] in mks units. Typically, the waves and Stokes Drift have an on-shelf component owing to the greater scope for generation provided by the off-shelf area of ocean. This transport is concentrated near the surface; it represents a difference (tracked water movement - average velocity at one point) rather than absolute circulation and is likely to be offset by opposing flow elsewhere in the water column.
Shelf edge topography only affects the longest waves. For example, with the criterion (wavenumber × depth) < 2, only waves of period > 14 s “feel” the bottom at 100 m depth.
Impacts
These include surface elevations and waves, especially at the coast. Circulation of water, and energy going into turbulence and mixing, all affect water properties and contents.
Surface elevations and waves
Theory and modelling suggest that sea-level variability on the longest spatial scales transmits from ocean to shelf-sea and coast [24][25]. Tides are a notable example. However, satellite altimetry shows a minimum of sea-level variance at the shelf edge, compared with the shelf sea and open ocean [26], yet coherence along the continental slope. This suggests that shelf seas and open oceans have separate causes of sea-level variations (e.g. weather and eddies respectively) but some inhibition to transmission across the continental slope. Weather over the shelf tends to generate coastal-trapped wave mode 1, which typically has a node near the shelf edge, and perhaps typical eddy scales are too short. This is the subject of continuing research.
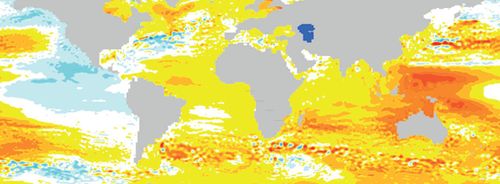
Sea level is rising globally and typical rates at the coast are similar to those in the open ocean now inferred from satellite altimetry. However, local sea level change can differ from the global average (figure 10). In the western tropical Pacific, sea level rise rates were up to 10 mm/yr averaged over 1993 to 2012 compared with a global mean of about 3 mm/yr. In contrast, sea level fell during this period in most of the east Pacific from Alaska to Peru. Varying winds, variability such as El Niño, oceanic thermal expansion and melted ice can alter ocean currents and associated sea level differences [27]. Ice re-distribution as water affects the earth’s gravitational field and hence “level”. These factors are all large-scale and are expected to transmit from ocean basins across the shelf to the coast. [Other factors are local or associated with land movement: water extraction, sediment compaction, tectonics, storms, earthquakes, landslides, uplift after the last ice age.]
The largest (and usually longest) surface waves are generated over a large “fetch” (extent of open ocean giving uninterrupted wind forcing). Hence they tend to come from deep ocean to shelf sea where they may have most impact: long waves steepen in shallower water; soft coasts and mobile sediments are vulnerable to stresses from wave motion.
Flushing / water renewal
These concepts are best applied to semi-enclosed seas with coasts bounding well-defined inflows and outflows. [In open sea areas, flushing and residence times can be arbitrarily shortened merely by decreasing the area in question.] However, these concepts help to assess the effectiveness of ocean-shelf transports. Thus around [math]42^{\circ}N[/math] off north-west Spain, average exchange across the 200m depth contour was estimated as [math]3.1 \; m^2/s[/math] [28], a large value and sufficient to replace the shelf–sea cross-section [math]3.32 \; km^2[/math] in only about 12 days. Estimates of Irish Sea outflow are similarly [math]2 - 3 \; m^2/s[/math] [i.e. mostly [math](0.7-1.1) \times 10^5 \; m^3/s[/math] through the North Channel width 35 km [29]]. However, this is only the volume of the Irish Sea in about one year (resupplied mostly by water originally from the Atlantic). Similarly inflows to the North Sea are 1.5-2 Sv mostly from the North [30], corresponding to [math]2 - 3 \; m^2/s[/math] on average across Scotland – Shetland – Norway. However, the North Sea is large and this inflow only amounts to the North Sea volume in about one year. Indeed, within the North Sea, the northern area tends to be flushed in a shorter time whereas in the centre the through-flow is much slower.
Turbulence and Mixing
As elsewhere, important processes for generating turbulence and consequent mixing near the continental shelf edge are: wind forcing and surface waves for surface mixing, internal tides and waves for mixing in the interior, tidal currents and internal waves near the bottom (e.g. Huthnance [3]). The intensity varies greatly according to context. At the Celtic Sea shelf edge, internal waves are very variable [31] but large, especially at (irregular) times near spring tides, and are associated with pycnocline mixing and density reduction near the bottom [32]. An upward flux of nitrate with a spring-neap cycle further demonstrates internal tidal forcing, associated with the passage of internal solitons around spring tides [33].
Biogeochemistry
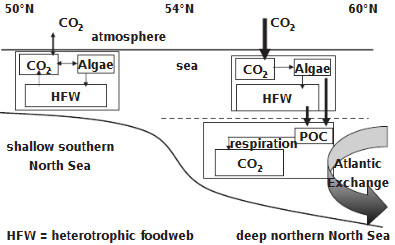
Many studies have discussed enhanced primary production on continental shelves in upwelling regions. These are typically at sub-tropical eastern ocean margins; winds with an equatorward component (at least seasonally) drive surface Ekman transport offshore. These surface waters are replaced by nutrient-rich waters from below, fuelling phytoplankton growth. Prevailing winds give the north-west European continental shelf overall down-welling circulation. Nevertheless, studies suggest a net supply of nitrate from the ocean to the North Sea [35] and to the west of Scotland [36]. Moreover, sinking of ensuing plankton leads to an off-shelf near-bottom carbon flux west of Scotland [36] as suggested more widely from Ireland to the Norwegian Trench on the basis of the down-welling circulation [37] [38].
Seasonally stratified shelf seas may act as sinks of atmospheric [math]CO_2[/math] (the shelf-sea carbon “pump”). Primary production takes up atmospheric [math]CO_2[/math]; sinking, sub-thermocline respiration of organic matter and off-shelf transport in a bottom layer follow before winter mixing gives re-exposure to the atmosphere (figure 11). Nitrate and carbon cycles over Goban Spur, as constructed in OMEX [39], also involve import of nitrate to the shelf from the deeper ocean and export of carbon from the shelf break to deeper on the continental slope. Distinctive mixing by internal tides and solitons at the Celtic Sea shelf edge, with the resulting upward nitrate flux, fuels a sub-surface chlorophyll maximum demonstrating consequent growth of phytoplankton. Compared with the adjacent Celtic (shelf) Sea and northeast Atlantic Ocean, shelf-edge vertical mixing of nitrate is enhanced and correspondingly the largest phytoplankton cells are found in surface waters there [33][40]. The shelf-edge internal tide (globally ubiquitous) is suggested to support shelf-edge fisheries by providing large-celled phytoplankton for first-feeding fish larvae without any need to coincide with a spring bloom; moreover large-cell phytoplankton favour particulate organic carbon export and sequestration in deep water and sediments [40], see Ocean carbon sink.
Further Reading
Huthnance JM, 1995. Circulation, exchange and water masses at the ocean margin: the role of physical processes at the shelf edge. Progress in Oceanography 35(4), 353-431.
Huthnance, J., Hopkins, J., Berx, B., Dale, A., Holt, J., Hosegood, P., Inall, M., Jones, S., Loveday, J.R., Miller, P.I., Polton, J., Porter, M. and Spingys, C. 2022. Ocean shelf exchange, NW European shelf seas: Measurements, estimates and comparisons. Progress in Oceanography 202, 102760
Johnson J, Chapman P (eds.), 2009-2011. Deep ocean exchange with the shelf. Ocean Science, Special Issue 18. http://www.ocean-sci.net/special_issue18.html
Journal of Marine Systems Volume 32, Issues 1–3 pp. EX1-EX2, 1-252 (April 2002): Exchange Processes at the Ocean Margins
Liu, K-K, Atkinson L, Quinones R, Talaue-McManus L (Eds.), 2010. Carbon and Nutrient Fluxes in Continental Margins: A Global Synthesis. Springer-Verlag Berlin Heidelberg, ISBN 978-3-540-92735-8. http://www.springer.com/us/book/9783540927341.
Robinson AR, Brink KH (editors). 1998. The Global Coastal Ocean. The Sea, Volumes 10, 11, 13, 14. John Wiley & Sons, New York. (1998, 1998, 2005, 2006 respectively).
Related articles
References
- ↑ Liu KK, Atkinson L, Quiñones RA, Talaue-McManus L, 2010. Biogeochemistry of Continental Margins in a Global Context. Pp3-24 in Carbon and Nutrient Fluxes in Continental Margins: A Global Synthesis (eds Liu KK, Atkinson L, Quiñones RA, Talaue-McManus L), Springer.
- ↑ Pauly D, Christensen V, Guenette Set al. 2002. Towards sustainability in world fisheries. Nature 418(6898), 689-695.
- ↑ 3.0 3.1 3.2 3.3 3.4 3.5 Huthnance JM, 1995. Circulation, exchange and water masses at the ocean margin: the role of physical processes at the shelf edge. Progress in Oceanography 35(4), 353-431.
- ↑ Robinson AR, Brink KH, Ducklow HW, Jahnke RA, Rothschild BJ, 2005. Interdisciplinary multiscale coastal dynamical processes and interaction. The Sea 13 (Robinson AR, Brink KH, eds.), 3-35.
- ↑ Joyce TM, 1991. Review of U.S. contributions to warm-core rings. Reviews of Geophysics, S29, 610-616.
- ↑ Neshyba SJ, Mooers CNK, Smith RL, Barber RT, 1989. Poleward flows along eastern ocean boundaries. Coastal and Estuarine Studies 34. Springer-Verlag New York, Inc. ISBN 0-387-97175-O. 374 pp.
- ↑ 7.0 7.1 Huthnance JM, Holt JT, Wakelin SL, 2009. Deep ocean exchange with west-European shelf seas. Ocean Science 5, 621-634, doi:10.5194/os-5-621-2009.
- ↑ Kara, AB, Wallcraft AJ, Metzger EJ, Hurlburt HE, Fairall CW, 2007. Wind stress drag coefficient over the global ocean. Journal of Climate 20, 5856-5864. DOI: http://dx.doi.org/10.1175/2007JCLI1825.1
- ↑ Green MO, McCave IN, 1995. Seabed drag coefficient under tidal currents in the eastern Irish Sea. Journal of Geophysical Research 100 (C8), 16057–16069. DOI: 10.1029/95JC01381
- ↑ Campbell NJ, 1964. The origin of cold high salinity water in Foxe Basin. Journal of Fisheries Research Board of Canada 21, 45–55.
- ↑ Ivanov VV, Shapiro GI, Huthnance JM, Aleynik DL, Golovin PN, 2004. Cascades of dense water around the world ocean. Progress in Oceanography 60, 47-98.
- ↑ Shapiro GI, Huthnance JM, Ivanov VV, 2003. Dense water cascading off the continental shelf. Journal of Geophysical Research 108(C12), 3390. doi:10.1029/2002JC001488.
- ↑ Prandle D, 1984. A modelling study of the mixing of 137Cs in the seas of the European continental shelf. Philosophical Transactions of the Royal Society of London A, 310, 407–436.
- ↑ Small J, Hornby B, Prior M, Scott J, 1998. Internal solitons in the ocean: prediction from SAR. At http://www.whoi.edu/science/AOPE/people/tduda/isww/text/small/jsmall.htm
- ↑ Vlasenko V, Stashchuk N, Inall ME, Hopkins JE, 2014. Tidal energy conversion in a global hot spot: On the 3‐D dynamics of baroclinic tides at the Celtic Sea shelf break. Journal of Geophysical Research: Oceans 119 (6), 3249-3265.
- ↑ Lien R-C, Tang TY, Chang MH, D’Asaro EA, 2005. Energy of nonlinear internal waves in the South China Sea. Geophysical Research Letters 32 (5). DOI: 10.1029/2004GL022012
- ↑ Garret CJR and Munk WH (1979) Internal Waves in the Ocean. Ann. Rev. Fluid Mech. 11, 339-369
- ↑ Huthnance JM, 1981. Waves and currents near the continental shelf edge. Progress in Oceanography 10, 193-226.
- ↑ Thorpe SA, 1992. The generation of internal waves by flow over the rough topography of a continental slope. Proceedings of the Royal Society of London, A439, 115-130.
- ↑ Chapman DC, Haidvogel DB, 1993. Generation of internal Iee waves trapped over a tall isolated seamount. Geophysical and Astrophysical Fluid Dynamics 69, 33-54.
- ↑ Hall RA, Huthnance JM, Williams RG, 2013. Internal wave reflection on shelf slopes with depth-varying stratification. Journal of Physical Oceanography 43, 248-258.
- ↑ Alford MH, Peacock T, MacKinnon JA, Nash JD, Buijsman MC, Centurioni LR, Chao S-Y, Chang M-H, Farmer DM, Fringer OB, Fu K-H, Gallacher PC, Graber HC, Helfrich KR, Jachec SM, Jackson CR, Klymak JM, Ko DS, Jan S, Johnston TMS, Legg S, Lee I-H, Lien R-C, Mercier MJ, Moum JN, Musgrave R, Park J-H, Pickering AI, Pinkel R, Rainville L, Ramp SR, Rudnick DL, Sarkar S, Scotti A, Simmons HL, St Laurent LC, Venayagamoorthy SK, Wang Y-H, Wang J, Yang YJ, Paluszkiewicz T, Tang T-Y, 2015. The formation and fate of internal waves in the South China Sea. Nature 521, 65-69.
- ↑ Kenyon KE, 1969. Stokes drift for random gravity waves. Journal of Geophysical Research 74, 6991-6994.
- ↑ Huthnance JM, 1987. Along-shelf evolution and sea levels across the continental slope. Continental Shelf Research 7, 957-974.
- ↑ Huthnance JM, 2004. Ocean‐to‐shelf signal transmission: A parameter study. Journal of Geophysical Research: Oceans 109, C12029, 11pp. doi:10.1029/2004JC002358.)
- ↑ Hughes CW, Meredith MP, 2006. Coherent sea-level fluctuations along the global continental slope. Philosophical Transactions of the Royal Society of London, A, 364 (1841). 885-901. 10.1098/rsta.2006.1744
- ↑ 27.0 27.1 Church JA, Clark PU, Cazenave A, Gregory JM, Jevrejeva S, Levermann A, Merrifield MA, Milne GA, Nerem RS, Nunn PD, Payne AJ, Pfeffer WT, Stammer D, Unnikrishnan AS, 2013: Sea Level Change. In: Climate Change 2013: The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change [Stocker TF, Qin D, Plattner G-K, Tignor M, Allen SK, Boschung J, Nauels A, Xia Y, Bex V, Midgley PM (eds.)]. Cambridge University Press, Cambridge, United Kingdom and New York, NY, USA.
- ↑ Huthnance JM, Van Aken HM, White M, Barton ED, Le Cann B, Coelho EF, Fanjul EA, Miller P, Vitorino J, 2002. Ocean margin exchange - water flux estimates. Journal of Marine Systems 32 (1-3), 107-137.
- ↑ Knight PJ, Howarth MJ, 1999. The flow through the north channel of the Irish Sea. Continental Shelf Research 19, 693-716.
- ↑ Otto L, Zimmerman JTF, Furnes G, Mork M, Saetre R, Becker G, 1990. Review of the physical oceanography of the North Sea. Netherlands Journal of Sea Research 26 (2), 161-238.
- ↑ Green JAM, Simpson JH, Legg S, Palmer MR, 2008. Internal waves, baroclinic energy fluxes and mixing at the European shelf edge. Continental Shelf Research 28, 937-950.
- ↑ Palmer MR, Stephenson GR, Inall ME, Balfour C, Düsterhus A, Green JAM, 2015. Turbulence and mixing by internal waves in the Celtic Sea determined from ocean glider microstructure measurements. Journal of Marine Systems 144, 57-69.
- ↑ 33.0 33.1 Sharples J, Tweddle JF, Green JAM, Palmer MR, Kim Y-N, Hickman AE, Holligan PM, Moore CM, Rippeth TP, Simpson JH, Krivtsov V, 2007. Spring-neap modulation of internal tide mixing and vertical nitrate fluxes at a shelf edge in summer. Limnology and Oceanography 52 (5), 1735-1747.
- ↑ Thomas H, Bozec Y, Elkalay K, de Baar HJW, 2004. Enhanced open ocean storage of CO2 from shelf sea pumping. Science 304, 1005-1008.
- ↑ Pätsch J, Kühn W, 2008. Nitrogen and carbon cycling in the North Sea and exchange with the North Atlantic—A model study. Part I. Nitrogen budget and fluxes. Continental Shelf Research 28 (6), 767-787.
- ↑ 36.0 36.1 Proctor R, Chen F, Tett PB, 2003. Carbon and nitrogen fluxes across the Hebridean shelf break, estimated by a 2D coupled physical-microbiological model. Science of the Total Environment 314, 787-800.
- ↑ Holt J, Wakelin S, Huthnance J, 2009. Down-welling circulation of the northwest European continental shelf: A driving mechanism for the continental shelf carbon pump. Geophysical Research Letters 36, L14602.
- ↑ Wakelin SL, Holt JT, Blackford JC, Allen JI, Butenschön M, Artioli Y, 2012. Modeling the carbon fluxes of the northwest European continental shelf: Validation and budgets. Journal of Geophysical Research Oceans 117 (C5), C05020. DOI: 10.1029/2011JC007402.
- ↑ Wollast R, Chou L, 2001. The carbon cycle at the ocean margin in the northern Gulf of Biscay. Deep-Sea Research II, 48 (14-15), 3265-3293.
- ↑ 40.0 40.1 Sharples J, Moore CM, Hickman AE, Holligan PM, Tweddle JF, Palmer MR, Simpson JH, 2009. Internal tidal mixing as a control on continental margin ecosystems. Geophys. Res. Lett., 36, L23603, doi:10.1029/2009GL040683.
- ↑ Fennel K, 2010. The role of continental shelves in nitrogen and carbon cycling: Northwestern North Atlantic case study. Ocean Science 6, 539-548.
Please note that others may also have edited the contents of this article.
|