Difference between revisions of "Shoreface profile"
Dronkers J (talk | contribs) |
Dronkers J (talk | contribs) |
||
| Line 1: | Line 1: | ||
{{Definition|title=Shoreface | {{Definition|title=Shoreface | ||
| − | |definition= The shoreface is the nearshore zone of the inner continental shelf that is bounded landward by the | + | |definition= The shoreface is the nearshore zone of the inner continental shelf that is bounded landward by the mean low-water line and that extends seaward to where the influence of wave action on sediment transport is on average minor compared to other influences. A similar definition is: The shoreface is the zone seaward of the shoreline where offshore generated waves interact with the upward sloping seabed. }} |
{{Definition|title=Shoreface profile | {{Definition|title=Shoreface profile | ||
| Line 22: | Line 22: | ||
where <math>H_{12h/y}</math> is the effective wave height just seaward of the breaker zone that is exceeded on average for 12 hours per year (i.e. the significant wave height with a probability of yearly exceedance of 0.137%) and <math>T_{12h/y}</math> the associated wave period. Later refinements of this formula are discussed in the article [[Closure depth]]. Typical values of <math>h_{in}</math> are in the range 3 – 10 m. The width of the upper shoreface typically ranges between about hundred meters and a few kilometers and the average slope between 1/10 and 1/200. The upper shoreface is sometimes also called breaker zone or surf zone, although broken onshore surfing wave bores usually only occur in the shoreward part of the upper shoreface. It is therefore preferable to use the designation upper shoreface as a fixed geographical indication, and the designations surf zone or breaker zone as a physical indication of the zone where broken waves surf onshore. This latter zone is narrow and close to the shoreline in a gentle wave climate and can be very wide under storm conditions, extending from the seaward boundary of the upper shoreface to the dunefoot. Other subdivisions of the coastal profile include the [[active coastal zone]], the beach face and the backshore, see [[Definitions of coastal terms]]. | where <math>H_{12h/y}</math> is the effective wave height just seaward of the breaker zone that is exceeded on average for 12 hours per year (i.e. the significant wave height with a probability of yearly exceedance of 0.137%) and <math>T_{12h/y}</math> the associated wave period. Later refinements of this formula are discussed in the article [[Closure depth]]. Typical values of <math>h_{in}</math> are in the range 3 – 10 m. The width of the upper shoreface typically ranges between about hundred meters and a few kilometers and the average slope between 1/10 and 1/200. The upper shoreface is sometimes also called breaker zone or surf zone, although broken onshore surfing wave bores usually only occur in the shoreward part of the upper shoreface. It is therefore preferable to use the designation upper shoreface as a fixed geographical indication, and the designations surf zone or breaker zone as a physical indication of the zone where broken waves surf onshore. This latter zone is narrow and close to the shoreline in a gentle wave climate and can be very wide under storm conditions, extending from the seaward boundary of the upper shoreface to the dunefoot. Other subdivisions of the coastal profile include the [[active coastal zone]], the beach face and the backshore, see [[Definitions of coastal terms]]. | ||
| − | The division between upper and lower shoreface profiles is often marked by an inflexion point, a bar or a terrace, indicating transition of morphodynamics <ref>Wright, L.D., Chappel, J., Thom, B.G., Bradshow, M.P. and Cowell, P. 1979. Morphodynamics of reflective and dissipative beach and inshore systems: south-eastern Australia. Mar.Geol. 32: 105-140</ref><ref>Niedoroda, A.W., Swift, D.J., Hopkins, T.S. and Ma, C.-M. 1984. Shoreface morphodynamics on wave-dominated coasts. Mar. Geol. 60: 331–354</ref><ref>Larson, M., Kraus, N.C. and Wise, R.A. 1999. Equilibrium beach profiles under breaking and non-breaking waves. Coast. Eng. 36: 59–85</ref> (Fig. 1). Another indicator of the transition between upper and lower shoreface is a break in the trend of the sediment grainsize with depth<ref>Aragones, L., Pagan, J.I., Lopez, I. and Serra, J.C. 2018. Depth of closure: New calculation method based on sediment data. International Journal of Sediment Research 33: 198-207</ref>. The shoreface | + | The division between upper and lower shoreface profiles is often marked by an inflexion point, a bar or a terrace, indicating transition of morphodynamics <ref>Wright, L.D., Chappel, J., Thom, B.G., Bradshow, M.P. and Cowell, P. 1979. Morphodynamics of reflective and dissipative beach and inshore systems: south-eastern Australia. Mar.Geol. 32: 105-140</ref><ref>Niedoroda, A.W., Swift, D.J., Hopkins, T.S. and Ma, C.-M. 1984. Shoreface morphodynamics on wave-dominated coasts. Mar. Geol. 60: 331–354</ref><ref>Larson, M., Kraus, N.C. and Wise, R.A. 1999. Equilibrium beach profiles under breaking and non-breaking waves. Coast. Eng. 36: 59–85</ref> (Fig. 1). Another indicator of the transition between upper and lower shoreface is a break in the trend of the sediment grainsize with depth<ref>Aragones, L., Pagan, J.I., Lopez, I. and Serra, J.C. 2018. Depth of closure: New calculation method based on sediment data. International Journal of Sediment Research 33: 198-207</ref>. The shoreface bordered on the landward side by the [[Definitions of coastal terms#Beach face|beach face]]. This is the zone where under average conditions waves collapse and run up the beach (see [[Swash zone dynamics]]). The upper shoreface profile is often undulated due to the presence of one or several bars, see [[Nearshore sandbars]]. These bars have mainly a shore parallel orientation, but more complex three-dimensional patterns are ubiquitous (see [[Rhythmic shoreline features]] and Fig. 2). |
Revision as of 10:20, 27 March 2021
Definition of Shoreface:
The shoreface is the nearshore zone of the inner continental shelf that is bounded landward by the mean low-water line and that extends seaward to where the influence of wave action on sediment transport is on average minor compared to other influences. A similar definition is: The shoreface is the zone seaward of the shoreline where offshore generated waves interact with the upward sloping seabed.
This is the common definition for Shoreface, other definitions can be discussed in the article
|
Definition of Shoreface profile:
The shoreface profile, often called beach profile, is the cross-shore coastal depth profile of the shoreface.
This is the common definition for Shoreface profile, other definitions can be discussed in the article
|
Contents
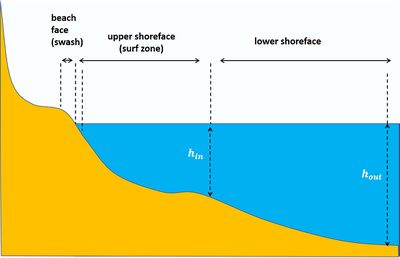
Introduction
In the literature one can find different definitions of the shoreface. The definition given here, which is most commonly used, follows the definition given in Stive and De Vriend (1995[1]). The shoreface can be divided in two zones, the upper shoreface and the lower shoreface. The upper shoreface is the zone where under average conditions waves break and the lower shoreface the zone where waves shoal. The lower part of the shoreface extends to the so-called outer closure depth [math]h_{out}[/math]; beyond this depth the seabed is hardly influenced by waves and wave-induced sediment transport is (on average) insignificant. The lower part of the shoreface is also called shoaling zone; wave shoaling is the process of wave amplification when waves travel from deep to shallow water before breaking (see Shallow-water wave theory). The outer closure depth [math]h_{out}[/math] has been empirically determined by Hallermeier (1981[2]) yielding the formula (see also Closure depth)
[math]h_{out} \approx (H_s – 0.3 \sigma) \, T_s \sqrt{\large\frac{g}{5000 d_{50}}\normalsize} \approx 0.013 H_s T_s \sqrt{\large\frac{g}{d_{50}}\normalsize} , \qquad (1)[/math]
where [math]H_s, T_s[/math] are the average significant wave height and period, [math]\sigma[/math] is the wave height standard deviation, [math]d_{50}[/math] the medium sediment grainsize and [math]g[/math] the gravitational acceleration. The second approximation in Eq. (1) follows from the assumption of a Rayleigh wave distribution (see Statistical description of wave parameters). Other criteria for the outer closure depth can be derived from the condition that the wave-induced bed shear stress should not exceed the threshold of sediment transport under extreme storms[3]. Typical values of the outer closure depth are in the range 10 – 30 m.
Most energy is dissipated in the upper part of the shoreface, where wave overturning and breaking occurs. The depth where waves start breaking depends on the wave climate and is therefore highly variable. A often used definition of the transition depth [math]h_{in}[/math] between upper and lower shoreface was also given by Hallermeier (1978[4]),
[math]h_{in} \approx 2.28 H_{12h/y}- 68.5 (\Large\frac{ H_{12h/y}^2}{g T_{12h/y}^2}\normalsize) , \qquad (2)[/math]
where [math]H_{12h/y}[/math] is the effective wave height just seaward of the breaker zone that is exceeded on average for 12 hours per year (i.e. the significant wave height with a probability of yearly exceedance of 0.137%) and [math]T_{12h/y}[/math] the associated wave period. Later refinements of this formula are discussed in the article Closure depth. Typical values of [math]h_{in}[/math] are in the range 3 – 10 m. The width of the upper shoreface typically ranges between about hundred meters and a few kilometers and the average slope between 1/10 and 1/200. The upper shoreface is sometimes also called breaker zone or surf zone, although broken onshore surfing wave bores usually only occur in the shoreward part of the upper shoreface. It is therefore preferable to use the designation upper shoreface as a fixed geographical indication, and the designations surf zone or breaker zone as a physical indication of the zone where broken waves surf onshore. This latter zone is narrow and close to the shoreline in a gentle wave climate and can be very wide under storm conditions, extending from the seaward boundary of the upper shoreface to the dunefoot. Other subdivisions of the coastal profile include the active coastal zone, the beach face and the backshore, see Definitions of coastal terms.
The division between upper and lower shoreface profiles is often marked by an inflexion point, a bar or a terrace, indicating transition of morphodynamics [5][6][7] (Fig. 1). Another indicator of the transition between upper and lower shoreface is a break in the trend of the sediment grainsize with depth[8]. The shoreface bordered on the landward side by the beach face. This is the zone where under average conditions waves collapse and run up the beach (see Swash zone dynamics). The upper shoreface profile is often undulated due to the presence of one or several bars, see Nearshore sandbars. These bars have mainly a shore parallel orientation, but more complex three-dimensional patterns are ubiquitous (see Rhythmic shoreline features and Fig. 2).
Morphodynamic feedback
Sediment transport on the shoreface depends on the local wave climate. By 'wave climate' is meant the description of incoming waves in terms of a statistical distribution of incident wave directions, wave heights and wave periods (see Statistical description of wave parameters). Sediment transport also depends on the characteristics of bed sediments and on the seabed bathymetry. For small wave incidence angles (close to the shore-normal direction) the relevant seabed bathymetry is the cross-shore shoreface profile.
The shoreface profile influences cross-shore sediment transport, but the inverse is also true: changes of the shoreface profile result from gradients in the cross-shore sediment transport. This is called morphodynamic feedback: for a given incident wave field the shoreface profile determines the transformation of the wave field on the shoreface and therefore the resulting sediment transport, whereas the resulting sediment transport determines the shoreface profile. As a result of this morphodynamic feedback, the shoreface profile tends to an equilibrium shape for a given stable wave climate. A dynamic equilibrium is reached when onshore directed sediment transport and offshore directed transport are equal on average over a period exceeding the morphodynamic adaptation time. This holds for alongshore uniform coasts; the equilibrium requirement for non-uniform coasts is a vanishing divergence of the long-term average total sediment transport vector (cross-shore and alongshore). It was realised already long ago that the shoreface of a sedimentary coast should not be regarded as a geologically inherited feature, but as the result of natural adaptation to modern hydrodynamic conditions and sediment supply[9][10]. In real field situations, a coast never assumes its equilibrium profile; it is always in a transient state due to fluctuations in the wave climate. The coastal profile deviates from the equilibrium profile, even in the hypothetical case of a stationary wave climate, due to the cyclical or quasi-cyclical migration of seabed structures along the profile (see Nearshore sandbars).
Knowing how to determine the equilibrium shoreface profile has great practical interest. It provides quantitative insight in the response of the shoreface to changes in the local wave climate by human and natural causes; such insight is important for testing and improving the effectiveness of measures to combat coastal erosion and shoreline retreat.
Bedforms and sediment sorting
Morphodynamic feedback involves not only shoreface profile development, but also sediment grainsize and bed roughness. Grainsize and bed roughness strongly influence sediment transport [11]. Inversely, sediment transport strongly influences the grainsize distribution of bed sediments. Bed roughness depends on the presence of bedforms (ripples, dunes), which in turn depend on sediment transport and sediment grainsize. Consequently, shoreface profile development, wave transformation on the shoreface, sediment transport, grainsize sorting and bedform emergence are all dynamically coupled processes.
Grainsize sorting is primarily due to differences in transport modes of different grainsize fractions. The finer fractions are more easily brought and maintained in suspension than the coarser fractions. They are transported further away from their initial location and tend to settle on the lower shoreface zone where wave-induced bed shear stresses are lower than on the upper shoreface. The coarse fraction is transported partly as suspended load and partly as bedload in the wave boundary layer. Wave transformation in shallow water leading to wave skewness (stronger onshore than offshore wave orbital velocity) and wave asymmetry (stronger onshore than offshore wave orbital acceleration) favour net onshore transport in the wave boundary layer. Fine sediments are resuspended from the upper shoreface due to undertow currents and turbulent shear stresses generated by breaking waves. Sediments on the upper shoreface are therefore coarser than on the lower shoreface. Fine sediment particles can still be present on the upper shoreface if they are 'hidden' by overlying coarser sediment particles – a phenomenon called 'bed armouring'.

The largest bedforms on the shoreface, such as shoreface-connected ridges (scale of several kilometers) and shore-oblique bars (scale of the order of hundred meters) are mainly related to morfodynamic feedback processes that interact with longshore transport, see the articles Sand ridges in shelf seas and Rhythmic shoreline features. Other large bedforms, the so-called nearshore sandbars or breaker bars (scale also of the order of 100 meters) arise from morphodynamic coupling with the wave breaking process, through the resulting flow structure at the breaker bar and interaction with cross-shore and longshore currents [12][13] - see Nearshore sandbars for a more detailed discussion. Bedforms at much smaller scales – ripples with wavelengths of the order of 1 m – are formed in the less exposed parts of the shoreface under low to moderate wave conditions. Morphodynamic feedback processes, leading to regular ripple patterns, involve ripple-induced near-bed flow circulation [14], vortex shedding at ripple crests [15] and non-linear ripple-ripple interactions [16]. For a more detailed discussion, see the articles Wave ripples and Wave ripple formation. The time lag of sediment suspension and settling related to vortex shedding at the ripple crests strongly influences the direction of residual cross-shore sediment transport on the shoreface, see section #Onshore wave-induced sediment transport.
Beach classification
The beach profile in the upper shoreface zone can be highly variable. The profile tends to steepen in periods of low-energy waves (especially long-period swell waves) and to flatten under high-energy storm waves (see Active coastal zone). Sediment characteristics also play an important role. Coarse-grained beaches, typical for high-energy coasts, have steeper slopes than fine-grained beaches, typical for moderate-to-low-energy coasts [17]. Gently sloping beaches dissipate almost completely the energy of incident waves, whereas steep-sloping beaches tend to reflect (partly) incident waves. For distinguishing between reflective and dissipative beaches the Dean-parameter [math]\Omega[/math] is often used [18][19]:
[math]\Omega = \Large \frac{H_s}{wT_p} \normalsize , \qquad(3)[/math]
where [math]H_s[/math] is the offshore significant wave height before breaking, [math]T_p[/math] is the peak spectral wave period and [math]w[/math] is the mean sediment fall velocity. Wright and Short (1984) [20] analysed beach profiles in Australia by evaluating the parameter [math]\Omega[/math], considering the local long-term average wave climate. They found that beach types could be classified as follows :
- reflective beaches correspond to [math]\Omega \lt 1 [/math];
- intermediate beaches correspond to [math]1\lt \Omega \lt 6 [/math];
- dissipative beaches correspond to [math]\Omega \gt 6 [/math].
The tidal range also influences the beach profile, because the surf zone is shifted across the shoreface with tidal level. For large tides (tidal range much larger than wave height) the surf zone covers during the tidal cycle a much larger area than for small tides. Beaches with large tides therefore have smaller upper shoreface slopes compared to beaches with the same [math]\Omega[/math] and small tides.
Another parameter that is often used for analysing the beach response to variations in the wave climate is the so-called surf similarity parameter, see Annex A.
Cross-shore sediment transport
Sediment transport on the shoreface depends on many processes. We focus on processes relevant for cross-shore transport. It should be realised, however, that longshore transport processes can also play an important role, for example by shifting the cross-shore position of the shoreface profile or by their role in the generation and evolution of nearshore sandbars[21]. See for example the articles: Natural causes of coastal erosion, Littoral drift and shoreline modelling and Rhythmic shoreline features. In the following we consider sandy beaches, with grain sizes typically between 0.2 and 0.5 mm (fine to medium sand). Coarse sedimentary beaches are dealt with in the article Gravel Beaches.
Onshore wave-induced sediment transport
Wave transformation in shallow water (see Shallow-water wave theory) is a major factor for onshore sediment transport [22]. Waves become skewed and asymmetric, producing near-bed shear stresses which are stronger in onshore direction than in offshore direction [23] [24][25]. Phase lags between bed shear stress and sediment suspension determine to which degree wave skewness induces onshore transport; in some situations (rippled bed, sheet flow) a large phase lag can reverse the net sediment transport direction. A large time lag implies that sediment particles that are stirred up by turbulent eddies during onshore orbital motion are transported offshore after reversal of the wave orbital velocity [26][27][23][28]. Onshore transport is stimulated by wave breaking that generates turbulent fluid motions and sediment resuspension under the wave crest [29]. The development of forward streaming at the top of the wave boundary layer also contributes to onshore transport [30], but this only holds for smooth beds and not in cases where the seabed is rippled, in cases of strongly skewed waves or for sheet flow conditions [31][32] (see also Sediment transport formulas for the coastal environment). Another, but generally lesser contribution to onshore sediment transport is further due to net mass transport in the upper layer between wave trough and wave crest.
Offshore wave-induced sediment transport
Offshore transport is due to so-called undertow: the compensating return current for the net onshore mass transport in the layer between wave trough and wave crest [33][34][35]. The undertow sand transport is particularly strong just in front of the location where waves are breaking and where much sediment is brought into suspension[36]. Bound infragravity waves may also contribute to net offshore sediment transport, as discussed in the article Infragravity waves.
Downslope sediment transport
Downslope transport occurs when sediment particles roll down the shoreface slope. However, this is not the primary cause of downslope sediment transport for fine to medium sandy beaches [37]. Gravity-induced sediment transport is a minor effect for two reasons: (1) the shoreface slope is small and (2) sediment particles are mainly transported in suspension, more than as bedload, especially on the upper shoreface. When a sediment particle is lifted from the bed to a certain level [math]z[/math] into the fluid, it moves with the wave orbital flow over a net distance [math]l[/math] until it settles again onto the bed. The net distance [math]l[/math] travelled by the sediment particle depends on the settling time; the longer the settling time, the greater the distance. The settling time is inversely proportional to the sediment fall velocity [math]w[/math] and the distance [math]z[/math] to the bottom. Because the shoreface has a seaward dipping slope, for an offshore moving sediment particle the distance to the bottom increases, so the net travelled distance by is larger than for a flat bottom. The opposite holds for an onshore moving sediment particle. The slope effect thus requires a correction to the net sediment transport over a flat bottom, which depends on the bed slope [math]\tan \beta[/math] and the fall velocity [math]w[/math]. Formulas for suspended sediment transport assume in general that the slope effect can be represented by a correction factor to the wave-induced transport components of the form [38]
[math](1- \alpha \, \tan \beta \Large \frac{u }{w} \normalsize ) , \qquad(4)[/math]
where [math]u[/math] the instantaneous wave orbital velocity, [math]w[/math] the sediment fall velocity and [math]\alpha[/math] an efficiency parameter. This suggests that the morphodynamics of the upper shoreface is not so much determined by the sediment fall velocity [math]w[/math], but rather by the ratio of [math]w[/math] and the shoreface slope [math]\tan \beta[/math]. This has been confirmed by laboratory experiments, in which an approximate correspondence was found between coastal profiles and an adjusted Dean parameter[39]
[math]\Omega_{\beta} = \Large \frac{H_s \tan \beta}{wT_p} \normalsize . \qquad(5)[/math]
Experiments by Hattori and Kawamata (1980[40]) with different profile slopes show that onshore transport dominates for [math]\Omega_{\beta} \lt 0.08[/math] and offshore transport dominates for [math]\Omega_{\beta} \gt 0.08[/math]. An equilibrium slope therefore corresponds to
[math]\Omega_{\beta} \sim 0.08 . \qquad(6)[/math]
Comparison with Eq. (3) shows that reflective beaches have a steep slope and dissipative beaches a gentle slope.
Although not proven, it is often assumed that the slope effect is a major factor compensating for net wave-induced onshore transport (sum of wave-induced onshore and offshore transport components) in situations close to equilibrium. This implies that the equilibrium shoreface slope [math]\tan \beta [/math] will be steeper (larger [math]\tan \beta [/math]) for a coarser seabed (large fall velocity [math]w[/math]) than for a finer sediment bed (small fall velocity [math]w[/math], smaller [math]\tan \beta [/math]). This is expressed by the correction factor (4) and is in general agreement with field observations. When averaging over the wave period (or, more generally, the wave climate), the bed-slope effect on the total net sediment transport can be related to the equilibrium bed slope [math]\tan \beta_{eq}[/math] by the correction factor [math](1 – \tan \beta / \tan \beta_{eq})[/math] [41].
Shoreface modelling
The processes involved in sediment transport on the shoreface, as described above, are complicated and not yet fully understood. Even the most sophisticated numerical morphodynamic models, like XBEACH [42], make use of approximate empirical formulations for several processes contributing to sediment transport and involve parameters that need to be tuned. Empirical formulas for wave-induced sediment transport on the shoreface that are often used in practice are given in the articles Sediment transport formulas for the coastal environment and Sand transport. These formulas are based on laboratory and field measurements for a large range of conditions. Rough estimates can be obtained with the empirical default parameters given in these articles. For better estimates it is necessary to tune these parameters to field data. A more detailed discussion of shoreface modelling is presented in the article Modelling coastal hydrodynamics.
Equilibrium shoreface profile (sandy beach)
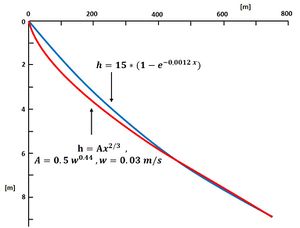
For a given stable wave climate the shoreface profile tends after a long period to an equilibrium profile. This equilibrium profile can be computed by applying sediment transport formulas to an initial profile for a period comprising all wave conditions according to local wave climate statistics. This requires heavy calculations that are moreover subject to large uncertainty margins. Therefore, equilibrium profiles derived from field observations are generally used. From analysis of a large number of beach profiles of the Californian and Danish coasts [43][44] and the US Atlantic and Gulf coasts [45] Bruun (1954) and Dean (1977) derived an equilibrium profile (often called the Dean/Bruun profile) of the form (Fig. 3)
[math]h(x)=A \; x^{2/3}, \qquad(7)[/math]
where [math]h[/math] is the water depth [m], [math]x[/math] the cross-shore distance [m] ([math]x=0[/math] at the shoreline) and [math]A [/math] a coefficient depending on the sediment fall velocity [math]w[/math] [m/s], [math] \quad A \approx 0.5 \; w^{0.44} [m^{1/3}][/math].
Dean [45] showed that the exponent 2/3 is consistent with the assumption of constant wave energy dissipation per unit volume throughout the surf zone.
Because the Bruun/Dean profile has an infinite slope at the shoreline, an alternative form was proposed by Bodge (1992) [46],
[math]h(x)=B \; (1 – e^{-kx}) , \qquad(8)[/math]
where [math]k[/math] is an empirical coefficient in the range [math]3 \; 10^{-5} - 1.16 \; 10^{-3} \; [m^{-1}][/math].
From an analysis of terrace-shaped shoreface profiles in northern Spain, Bernabeu et al. (2003) [47] found that the Bruun/Dean profile was adequate only for the upper shoreface; for the lower shoreface they proposed the form
[math]h(x)=A' \; (x-x_0)^{2/3} , \qquad(9)[/math]
where [math]x_0[/math] is determined such that the two profiles (7) and (9) match at the inflexion point between the upper and lower shoreface.
Inman et al. (1993) [48] used a similar procedure for the analysis of beach profiles for the coasts of south California, North Carolina and the Nile delta. They found best fits with exponents ranging between 0.3 and 0.45 instead of 2/3.
The cross-shore profile of gravel beaches is not the same as for sandy beaches, see: Gravel Beaches.
Analytical equilibrium models
Because of the earlier mentioned difficulties to derive model estimates of the equilibrium shoreface profile, taking into account the full wave climate, analytical models have been developed in which the wave climate is replaced by a single representative monochromatic incident wave. An alongshore uniform shoreface is assumed with perpendicular wave incidence. In these models a simple wave transformation model is used based on depth saturation of broken waves (wave height limited by depth) and shallow-water wave theory. An analytical expression for the equilibrium shoreface slope can be derived by requiring that the total wave-integrated cross-shore sediment flux equals zero throughout the shoreface zone [49] [37] [50]. Although these models reproduce certain properties also observed in the field, such as increasing profile steepness with increasing grain size and with increasing wave period, a more detailed comparison of computed profiles with observations exhibits large discrepancies. The computed concavity of the upper shoreface profile is much stronger than generally observed in the field and much stronger than for the Bruun/Dean profile. This is perhaps not surprising in view of the simplicity of the model for wave-induced sediment transport, which ignores many of the processes described in the previous paragraphs. These analytical models also ignore the influence of extreme storms, which can flatten the profile out to great depths within a few days. The profile adaptation time scale for the entire shoreface being quite long (order of centuries [37]), it is plausible that observed shoreface profiles generally correspond to transient profiles [50].
Annex A: Surf similarity parameter
Characteristic features that play a major role in the wave hydrodynamics of the breaker zone are the seabed slope [math]\tan \beta[/math], the still water depth [math]h[/math], the incident wave height [math]H_s[/math] and the wave period [math]T[/math], where all quantities refer to a location just seaward of the breakpoint. Battjes (1974[51]) has shown, however, that important processes depend mainly on a single parameter [math]\xi[/math], generally called surf similarity parameter or Iribarren parameter:
[math]\xi = \Large\frac{\tan \beta}{\sqrt{H_s/L}}\normalsize , \qquad (A1)[/math]
where [math]L=\Large\frac{g T^2}{2 \pi}\normalsize[/math] is the wavelength at the seaward boundary of the breaker zone and [math] H_s/L[/math] the wave steepness at this location. Using the wave breaking criterion for the surf zone [math]H_s/h =\gamma[/math] (breaker index) and the shallow-water wave celerity [math]c \approx \sqrt{gh}[/math], Eq. (A1) can be expressed as
[math]\xi \approx \Large \sqrt{\frac{\gamma}{2 \pi}}\, \frac{\tan \beta}{H_s /L}\normalsize . \qquad (A2)[/math]
The surf similarity parameter thus represents approximately the ratio of the seabed steepness and the steepness of the breaking wave. Experiments show that [math]\xi[/math] is also related to the breaker type: for [math]\xi \gt 2[/math] a breaker is collapsing or surging, for [math]0.4 \lt \xi \lt 2[/math] a breaker is plunging and for [math]\xi \lt 0.4 [/math] a breaker is spilling, where the values of [math]H_s[/math] and [math]L[/math] are to be taken at the breakpoint. It further appears that the breaker index [math]\gamma[/math] depends on [math]\xi[/math], that the wave reflection coefficient depends on [math]\xi[/math], and that the relative wave run-up [math]R/H_s[/math] is approximately proportional to [math]\xi[/math].
Related articles
- Nearshore sandbars
- Bruun rule
- Active coastal zone
- Coastal Hydrodynamics And Transport Processes
- Modelling coastal hydrodynamics
- Coastal and marine sediments
- Gravel Beaches
Further reading
Komar, P.D. 1998. Beach processes and sedimentation. Prentice Hall, London, pp. 544.
Woodroffe, C.D. 2002. Coasts, form, processes and evolution. Cambridge Univ.Press, 623 pp.
Dronkers, J. 2017. Dynamics of Coastal Systems. World Scientific Publ. Co. 753 pp.
References
- ↑ Stive, M.J.F. and De Vriend, H.J. 1995. Modelling shoreface profile evolution. Mar. Geol. 126: 235–248
- ↑ Hallermeier, R.J. 1981. A profile zonation for seasonal sand beaches from wave climate. Coast. Eng. 4: 253–277
- ↑ Valiente, N.G., Masselink, G., Scott, T., Conley, D. and McCarroll, R.J. 2019. Role of waves and tides on depth of closure and potential for headland bypassing. Mar. Geol. 407: 60–75
- ↑ Hallermeier, R.J. 1978. Uses for a calculated limit depth to beach erosion. In: 16th Coastal Engineering Conference, American Society of Civil Engineers, pp. 1493- 1512
- ↑ Wright, L.D., Chappel, J., Thom, B.G., Bradshow, M.P. and Cowell, P. 1979. Morphodynamics of reflective and dissipative beach and inshore systems: south-eastern Australia. Mar.Geol. 32: 105-140
- ↑ Niedoroda, A.W., Swift, D.J., Hopkins, T.S. and Ma, C.-M. 1984. Shoreface morphodynamics on wave-dominated coasts. Mar. Geol. 60: 331–354
- ↑ Larson, M., Kraus, N.C. and Wise, R.A. 1999. Equilibrium beach profiles under breaking and non-breaking waves. Coast. Eng. 36: 59–85
- ↑ Aragones, L., Pagan, J.I., Lopez, I. and Serra, J.C. 2018. Depth of closure: New calculation method based on sediment data. International Journal of Sediment Research 33: 198-207
- ↑ Cornaglia, P. 1889. Delle Spiaggie. Accademia Nazionale dei Lincei, Atti. Cl. Sci. Fis., Mat. e Nat. Mem. 5: 284-304
- ↑ Johnson, D.W. 1919. Shore processes and shoreline development. Prentice Hall, N-Y, 584 pp.
- ↑ Van Rijn, L.C. 1998. Principles of Coastal Morphology. Aqua Publications, The Netherlands (aqua publications.nl.
- ↑ Reniers, A.J.H.M., Thornton, E.B., Stanton, T.P. and Roelvink, J.A. 2004. Vertical flow structure during Sandy Duck: observations and modelling. Coast.Eng. 51: 237-260
- ↑ Jacobsen, N.G. and Fredsoe, J. 2014. Formation and development of a breaker bar under regular waves. Part 2: Sediment transport and morphology. Coastal Eng. 88: 55-68
- ↑ Sleath, J.F.A. 1984. Sea bed mechanics. Wiley, New York.
- ↑ Fredsøe, J., Andersen, K.H. and Sumer, M.B. 1999. Wave plus current over a ripple-covered bed. Coastal Eng. 38: 177-221
- ↑ Marieu, V., Bonneton, P., Foster, D.L. and Ardhuin, F. 2008. Modeling of vortex ripple morphodynamics. J. Geophys. Res. 113, C09007, doi:10.1029/2007JC004659
- ↑ Bujan, N., Cox, R. and Masselink, R. 2019. From fine sand to boulders: Examining the relationship between beach-face slope and sediment size. Marine Geology 417, 106012
- ↑ Gourlay, M. R. 1968. Beach and dune erosion tests. Rep. m935/m936, Delft Hydraul. Lab., Delft
- ↑ Dean, R.G. 1973. Heuristic models of sand transport in the surf zone. Proc. Conf. Eng. Dynamics in the Surf Zone, Sydney: 208-214.
- ↑ Wright, L.D. and Short, A.D. 1984. Morphodynamic variability of surf zones and beaches: a synthesis. Mar.Geol. 56: 93-118
- ↑ Walstra, D.J.R., Reniers, A.J.H.M., Ranasinghe, R., Roelvink, J.A. and Ruessink, B.G. 2012. On bar growth and decay during inter-annual net offshore migration. Coast Eng. 60: 190–200
- ↑ Elgar, S., Gallagher, E.L. and Guza, R.T. 2001.Nearshore sandbar migration. J.Geophys.Res. 106: 11,623-11,727
- ↑ 23.0 23.1 Nielsen, P. 1992. Coastal bottom boundary layers and sediment transport. In: Advanced Series on Ocean Engineering, IV. World Scientific.
- ↑ Abreu, T., Silva, P.A., Sancho, F. and Temperville, A. 2010. Analytical approximate wave form for asymmetric waves. Coastal Eng. 57: 656-667
- ↑ Van der A, D.A., O’Donoghue, T., Davies, A.G. and Ribberink, J.S. 2011. Experimental study of the turbulent boundary layer in acceleration-skewed oscillatory flow. J. of Fluid Mech. 684: 251-283
- ↑ Vincent, C.E. and Green. M.O. 1990. Field measurements of the suspended sand concentration profiles and fluxes and of the resuspensionm coefficient over a rippled bed. J.Geophys.Res. 95: 11591-11601
- ↑ Ribberink, J.S. and Al-Salem, A.A. 1995. Sheet flow and suspension of sand in oscillatory boundary layer. Coastal Eng. 25: 205-225
- ↑ Ruessink, B.G., Michallet, H., Abreu, T., Sancho, F., van der A, D.A., van der Werf, J.J. and Silva, P.A., 2011. Observations of velocities, sand concentrations, and fluxes under velocity-asymmetric oscillatory flows. J. Geophys. Res. 116, C03004, doi:10.1029/2010JC006443
- ↑ Christensen, D.F., Brinkkemper, J., Ruessink, G. and Aagaard, T. 2019. Field observations of intra-wave sediment suspension and transport in the intertidal and shallow subtidal zones. Marine Geology 413: 10–26
- ↑ Longuet-Higgins, M.S. 1953. Mass transport in water waves. Royal Soc. London, Phil.Trans. 245A: 535-581
- ↑ Trowbridge, J.H. and Madsen, O.S. 1984. Turbulent wave boundary layers: 2. Second-order theory and mass transport. J.Geophys.Res. 89: 7999-8007
- ↑ Kranenburg, W. M., Ribberink, J. S., Uittenbogaard, R. E. and Hulscher, S. J. M. H. 2012. Net currents in the wave bottom boundary layer: on wave shape streaming and progressive wave streaming. J. Geophys. Res. 117(F03005), doi:10.1029/2011JF002070
- ↑ Stive, M.J.F. and Wind, H.G. 1986. Cross-shore mean flow in the surf zone. Coastal Eng. 10: 325-340
- ↑ Cox, D.T. and Kobayashi, N. 1998. Application of an undertow model to irregular waves on plane and barred beaches. J.Coast.Res. 14: 1314-1324
- ↑ Gallagher, E.L., Elgar, S. and Guza, R.T. 1998. Observations of sand bar evolution on a natural beach. J. Geophys. Res. 103: 3203–3215
- ↑ Reniers, A.J.H.M., Thornton, E.B., Stanton, T.P. and Roelvink, J.A. 2004. Vertical flow structure during Sandy Duck: observations and modelling. Coast.Eng. 51: 237-260
- ↑ 37.0 37.1 37.2 Stive, M. J. F. and de Vriend, H. J. 1995. Modelling shoreface profile evolution. Marine Geology 126: 235–248
- ↑ Bagnold, R.A. 1963. Mechanics of marine sedimentation. In: The sea, vol.3. Ed. M.N.Hill, Wiley-Interscience: 507-528
- ↑ Bayle, P.M., Beuzen, T., Blenkinsopp, C.E., Baldock, T.E. and Turner, I.L. 2021. A new approach for scaling beach profile evolution and sediment transport rates in distorted laboratory models. Coastal Engineering 163, 103794
- ↑ Hattori, M. and Kawamata, R. 1980. Onshore–offshore transport and beach profile change. In: Proc. 17th ASCE Coastal Engineering Conference, 2, pp. 1175–1193
- ↑ Patterson, D.C. and Nielsen, P. 2016. Depth, bed slope and wave climate dependence of long term average sand transport across the lower shoreface. Coastal Engineering 117: 113–125
- ↑ https://oss.deltares.nl/web/xbeach/home
- ↑ Bruun, P. 1954. Coast erosion and the development of beach profiles. Beach Erosion Board, US Army Corps of Eng., Tech.Mem. 44: 1-79
- ↑ Dean, R.G. 1977. Equilibrium beach profiles: US Atlantic coast and Gulf coasts. Ocean Eng.Tech.Rep. 12, Univ. of Delaware, Newark, 45 pp .
- ↑ 45.0 45.1 Dean, R.G. 1977. Equilibrium beach profiles: US Atlantic coast and Gulf coasts. Ocean Eng.Tech.Rep. 12, Univ. of Delaware, Newark, 45 pp.
- ↑ Bodge, K.R. 1992. Representing equilibrium beach profiles with an exponential expression. J. Coastal Research 8: 47-55.
- ↑ Bernabeu, A.M., Medina, R. and Vidal, C. 2003. A morphological model of the beach profile integrating wave and tidal influences. Mar.Geol. 197: 95-116
- ↑ Inman, D.L., Elwany, M.H. and Jenkins, S.A. 1993. Shorerise and bar-berm profiles on ocean beaches. J.Geophy.Res. 98: 18181-18199
- ↑ Bowen, A.J. 1980. Simple models of nearshore sedimentation; beach profiles and longshore bars. In: The coastline of Canada, Ed. by S.B. McCann, Geological Survey of Canada, Ottawa, pp. 1-11
- ↑ 50.0 50.1 Ortiz, A.C. and Ashton, A.D. 2016. Exploring shoreface dynamics and a mechanistic explanation for a morphodynamic depth of closure. J. Geophys. Res. Earth Surf 121: 442–464 doi:10.1002/2015JF003699
- ↑ Battjes, J.A. 1974. Surf similarity. Proceedings 14th International Conference on Coastal Engineering, pp. 466–480
Please note that others may also have edited the contents of this article.
|